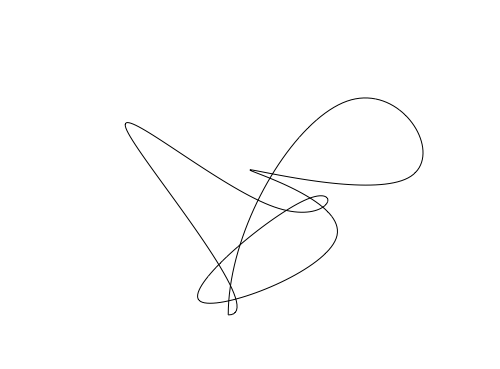
Knot or not?
The topological game involves a projection of a knot, drawn onto paper, such as:
Player 1 picks an intersection and assigns a crossing (which segment of the curve is "above" and which "below" the other segment) and marks it on the drawing (for instance by making bold the "top" segment). Then Player 2 picks another intersection and likewise assigns a crossing. The players alternate until every crossing is assigned. Player 1's goal is to create the trivial knot (or "un-knot" or simple loop) while Player 2 tries to make any other knot.
When you're done, ask the child to test the final answer with a piece of string tied in a loop.
Even if the child doesn't quite know what strategy to employ for "winning," after the crossings have been assigned you can ask the child to "figure out" or "guess" whether the string would create a knot or not. This makes the activity more like an interactive arts and crafts exercise, with the ensuing delight of finding whether the string forms a knot. Pulling the string tight I imagine the child shouting in glee: "KNOT!" (or "NOT!")
(Start with a few very simple projections. Later, let the child draw the projection.)