Return to Answer

To simplify, we can solve the constraint $\alpha \log a + (1-\alpha)\log (1-a)$ for $\alpha$: $$\alpha = \frac{L - \log(1-a)}{\log(a) - \log(1-a)}$$ and now rewrite our minimization as: minimize $$f(a) = \frac{(L - \log(1-a))(1-a)^2 + (\log(a)-L)a^2}{\log(a)-\log(1-a)}$$ over $$a \in (0, \min(e^L, 1-e^L)), \quad n\alpha \in \mathbb{N}$$

To simplify, we can solve the constraint $\alpha \log a + (1-\alpha)\log (1-a)$ for $\alpha$: \begin{equation} \tag{3} \alpha = \frac{L - \log(1-a)}{\log(a) - \log(1-a)} \end{equation} and now rewrite our minimization as: minimize $$f(a) = \frac{(L - \log(1-a))(1-a)^2 + (\log(a)-L)a^2}{\log(a)-\log(1-a)}$$ over $$a \in (0, \min(e^L, 1-e^L)), \quad n\alpha \in \mathbb{N}$$

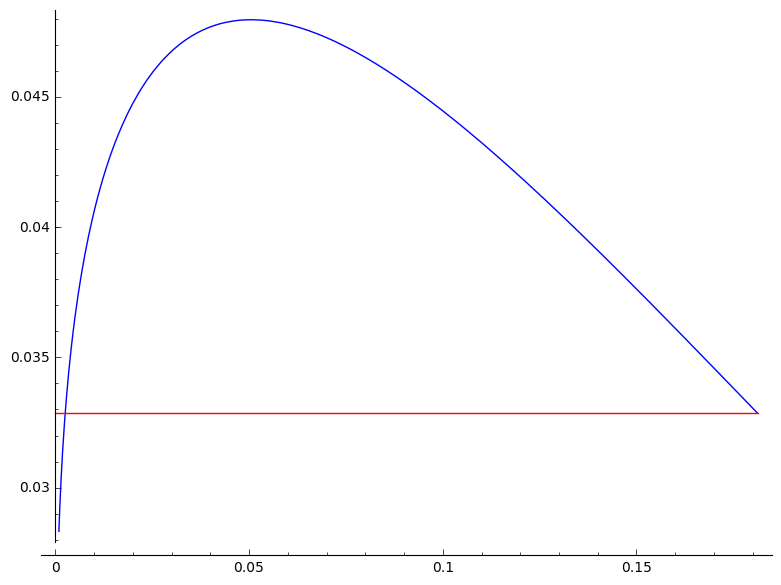
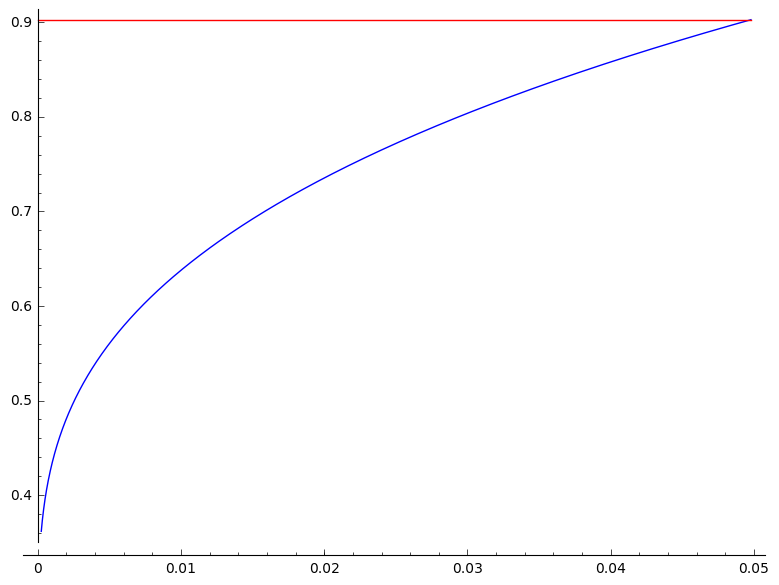
Edit: Here are some pictures for why the smallest value of $a$ below does always corresponds to the smallest value of $\alpha$. I think it's helpful to visualize first. Here is $\alpha$ as a function of $a$ for $L = \log(.99)$, $L = \log(.51)$, $L = \log(1/2)$, and $L = \log(.49)$.

$L = \log(.99)$ 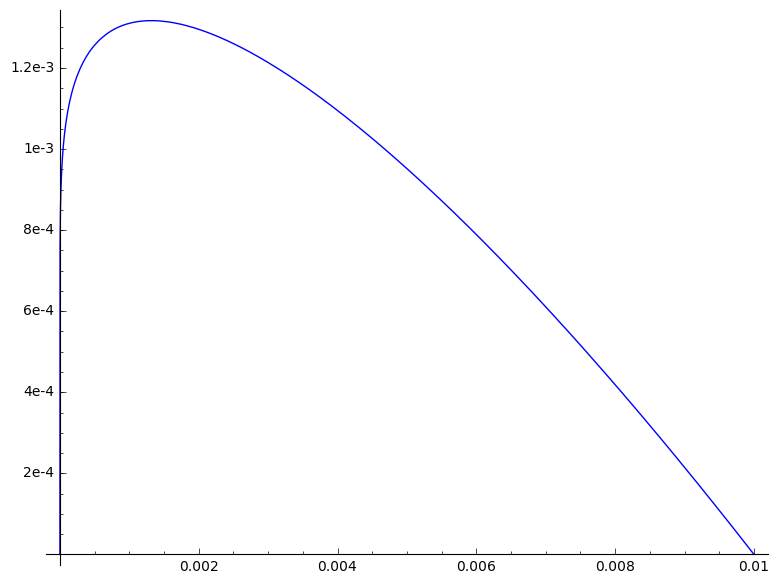$L = \log(.51)$ 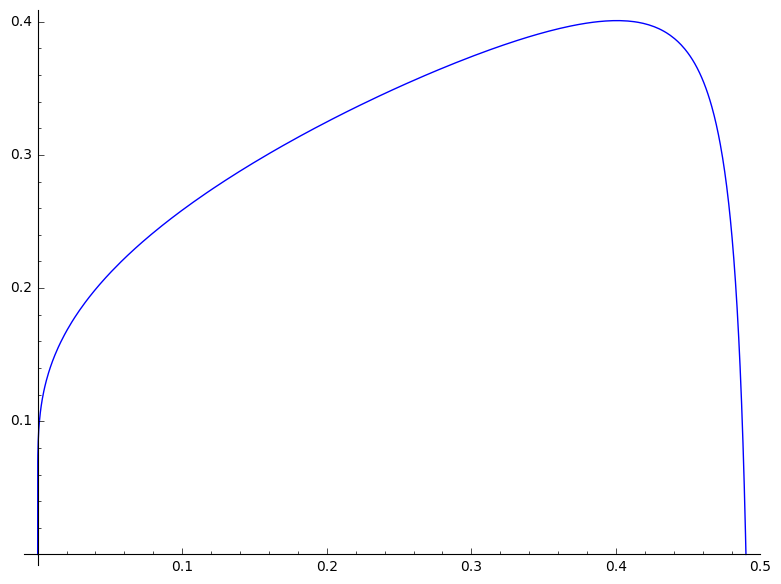$L = \log(.5)$ 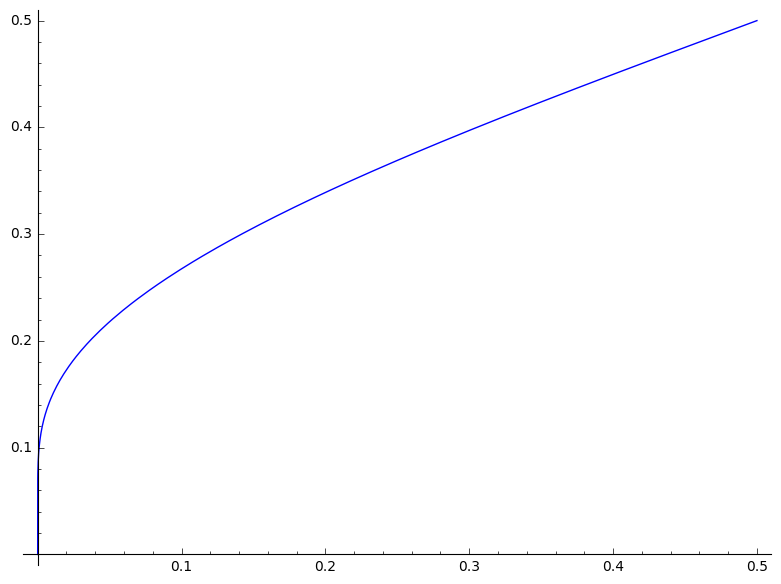$L = \log(.49)$

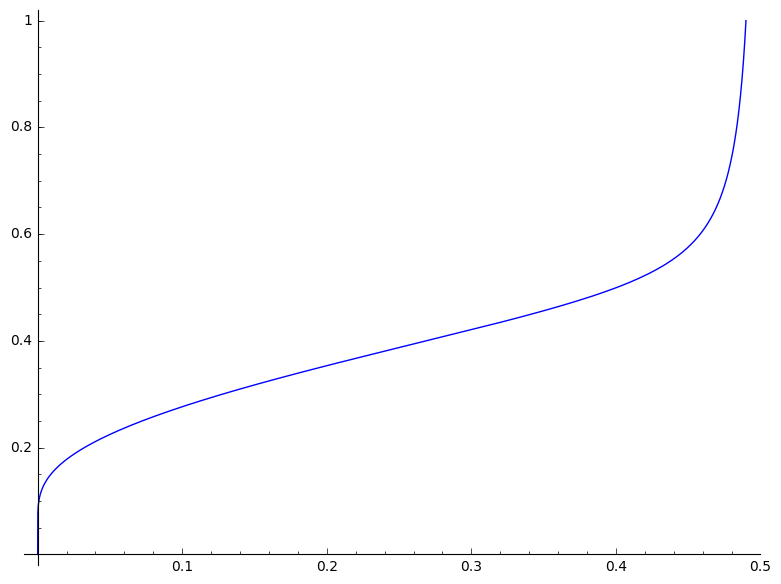
If $L \leq \log(1/2)$ then $\alpha$ as a function of $a$ has no critical points and is increasing (similar reasoning as with $f(a)$). Therefore taking the smallest possible $\alpha$ gives you the smallest possible $a$.

If $L > \log(1/2)$ then for $a \in [0, 1-e^{L}] = [0, min(e^L, 1-e^L)]$, $\alpha$ has a single critical point, a maximum, and is zero at the endpoints. Therefore, we can (mistakenly) choose $\alpha = 1/1000$ and then take $a$ to be large. But, you can visualize all allowable values of $a$ by drawing a discrete collection of horizontal lines on this picture (here's $L = log(.51), n=10$): 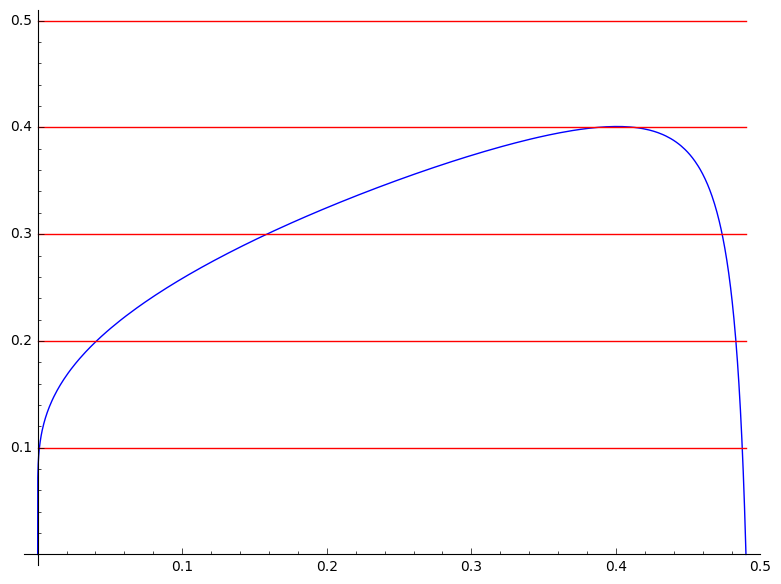The possible choices of $a$ in our discrete set are given by intersections of the red lines with our blue curve, by the nature of the function each red line has two intersections with the blue curve. The lowest red line has both the smallest and largest value of $a$. This picture also illustrates how to find the counterexample to your stackexchange question.

• For $s \geq 1$ we always take the matrix with diagonals $s^{1/n}$.
• For $s < 1$ and for $n$ large enough enough we have two possibilities. For $s$ large enough we take (still) the matrix with diagonals $s^{1/n}$. For $s$ small enough we instead solve $$a(1-a)^{n-1} = s$$ and take the smaller of the (at most two) solutions. The optimizer will be the matrix with one diagonal $a$ and the rest $1-a$. (Note for $s$ small enough we will have $a \approx s$ and $dist \approx 1$.

Given these three facts we see that if we forget the condition $n \alpha \in \mathbb{N}$ from our constraints we see that the infimum of $f$ is $0$ which is not realized for $a > 0$. The function either looks like or (These are L = -1/5 and -3.) The red line in these pictures is the value from taking the matrix of diagonals, (e^L - 1)^2.

• For $s \geq 1$ we always take the matrix with diagonals $s^{1/n}$.
• For $s < 1$ and we have two possibilities. For $n$ small enough we take (still) the matrix with diagonals $s^{1/n}$. For $n$ large enough we instead solve $$a(1-a)^{n-1} = s$$ and take the smaller of the (at most two) solutions. The optimizer will be the matrix with one diagonal $a$ and the rest $1-a$. (Note for $s$ small enough we will have $a \approx s$ and $dist \approx 1$.

Given these three facts we see that if we forget the condition $n \alpha \in \mathbb{N}$ from our constraints we see that the infimum of $f$ is $0$ which is not realized for $a > 0$. The function $f(a)$ either looks like or (These are L = -1/5 and -3.) The red line in these pictures is the value from taking the matrix of diagonals, (e^L - 1)^2.

Add-on: I will write what I know about $f(a)$ more precisely.

• For $L \geq \log (1/2)$ there is a solution to the constraint $\alpha \log a + (1-\alpha) \log (1-a) = L$ with $a= \alpha$. Equivalently, for $1/2 \leq y < 1$ there is a solution to $\frac{a^a}{(1-a)^{1-a}} = y$. There are actually two solutions on $[0, 1]$ related by $a_1 = 1-a_2$, so since we enforce $a<1/2$ there is only one solution on our domain for $a$.

• For $L < \log (1/2)$ there is no solution with $a = \alpha$.

• This means that $f$ as a function of $a$ has a critical point if $L \geq \log(1/2)$, namely where $a = \alpha$. If $L \leq \log(1/2)$ there is no critical point.

Checking these facts I checked items 2 and 3 with a CAS. For item 1 I did the following. First implicitly differentiate the constraint (1) with respect to $a$ to find $$ \frac{d\alpha}{da} \left(\log a - \log(1-a) \right) = - \left(\frac{\alpha}{a} \frac{1-\alpha}{1-a} \right) $$ Then differentiate $f$ and set it to zero to find $$ 2 (a - \alpha) \left(\log a - \log(1-a) \right) = (-2a + 1) \left( \frac{\alpha}{a} - \frac{1-\alpha}{1-a} \right) $$ Multiply by $a(1-a)$ to find $$ 2a(1-a)(a-\alpha) \left( \log a - \log(1-a) \right) = (-2a+1) \left(\alpha - a \right) $$ If $\alpha \neq a$ we can divide by $\alpha-a$ and find (checking that there's only one solution) $a = 1/2$. This is disallowed by our constraints (2). On the other hand, we have a solution if $\alpha = a$. Then, check that there is at most one solution to (1) with $\alpha = a$.

Checking these facts I checked items 2 and 3 with a CAS. For item 1 I did the following. First implicitly differentiate the constraint (1) with respect to $a$ to find $$ \frac{d\alpha}{da} \left(\log a - \log(1-a) \right) = - \left(\frac{\alpha}{a} - \frac{1-\alpha}{1-a} \right) $$ Then differentiate $f$ and set it to zero to find $$ 2 (a - \alpha) \left(\log a - \log(1-a) \right) = (-2a + 1) \left( \frac{\alpha}{a} - \frac{1-\alpha}{1-a} \right) $$ Multiply by $a(1-a)$ to find $$ 2a(1-a)(a-\alpha) \left( \log a - \log(1-a) \right) = (-2a+1) \left(\alpha - a \right) $$ If $\alpha \neq a$ we can divide by $\alpha-a$ and find (checking that there's only one solution) $a = 1/2$. This is disallowed by our constraints (2). On the other hand, we have a solution if $\alpha = a$. Then, check that there is at most one solution to (1) with $\alpha = a$.