Interesting rational constant concerning base-10 normal numbers.
Let $a$ be a real number with a base - 1010 decimal representation representation $a_1a_2\ldots a_n \ldots$ Denote the number of ways to write write $a_n$ as the sum of positive integers as $p(a_n)$ - also called the partition of the partition of $a_n$. I write $$\beta(a)=\sum_{n=1}^{\infty}{p\left(a_n\right)\above 1.1pt a_n}$$ If$A(n)=\sum_{k=1}^n p(a_k)$ and $B(n)= \sum_{k=1}^n a_k$ and set $$\beta(a)=\lim_{n\to \infty}{A(n)\above 1.5 pt B(n)}$$ If $a$ is a base-10 normal number then $\beta(a)={97 \above 1.5 pt 45}$ .
Note the converse of the above statement is false!
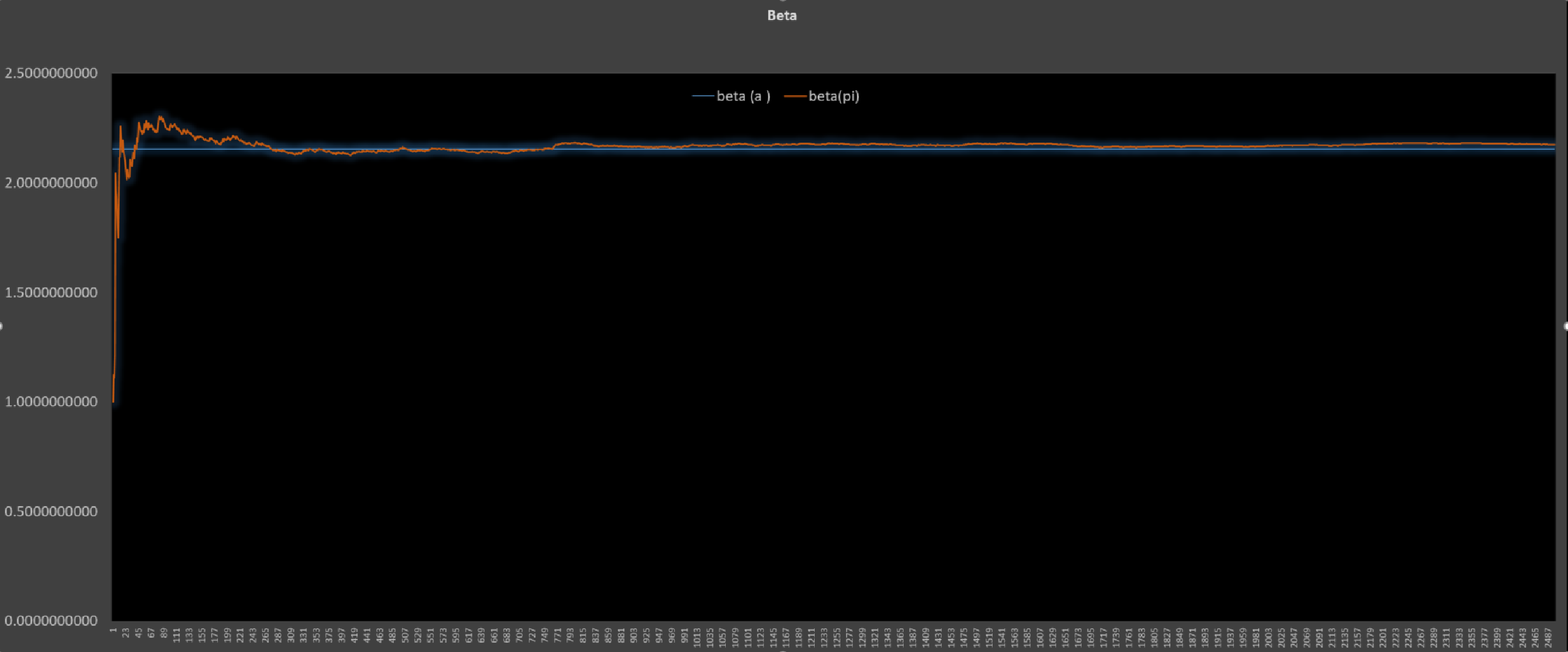
Numerically ${97\above 1.5pt 45}$ can be written $2.15\ldots$. If we believe $\pi$ is normal then we would expect $\beta(\pi)={97 \above 1.5 pt 45}$. Up to the $500\text{ }000$-th digit $\beta(\pi)=2.153781\ldots$ See the plot of $\beta(\pi)$ below. Note the blue line is the constant value ${97\above 1.5 pt 45}$ and the orange "graph" are the values of $\beta(\pi)$.