in Section 1 of loc. cit. a proof is given that there exists a graph $G$ and an automorphism $\alpha$ of $G$ as an abstract graph, such that there does not exist any embedding of (the geometric realization $\lvert G\rvert$ of ) $G$ into $\mathbb{R}^3$ such that $\alpha$ could be realized by an element of the group of leaving-$\lvert G\rvert$-invariant-as-a-point-set diffeomorphisms of $\mathbb{R}^3$. Note that in this rendition I rendered the author's "group of homeomorphisms of $G$ up to isotopy" into what to me evidently seems equivalent and clearer "automorphism of $G$ as an abstract graph". The example graph used by the author is $G:=$complete graph with six vertices.
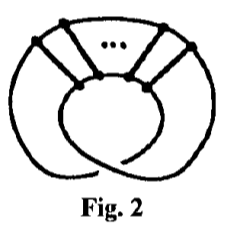
in Section 2 of loc. cit., first a proof in classical logic is given that for any embedding $\eta\colon M_r\to S^3$ of the $r$-rung Möbius ladder $M_r$ into the $3$-sphere $\mathbb{S}^3$, any orientation-reversing diffeomorphism $\varphi\colon S^3\to S^3$ has the property that if it fixes1 $\mathrm{im}(\eta)$ as a pointset, then $\varphi$ does not fix at least $r-2$ of the $r$ rungs of $M_r$. This is, partly, an interesting counterfactural (since the author later gives a proof that for an odd number of rungs, such $\varphi$ are impossible): roughly, if there is a symmetry of the Euclidean-space-embedded Möbius ladder, then it must needs jumble almost all the ladder's rungs. This implication is then put to use to give a proof that for an odd number of $r$ungs, such a $\varphi$ is impossible. Roughly: you cannot reverse the orientation of an embedded odd-rung-number Möbius ladder graph by a Euclidean symmetry. The author then gives an example that for even number of rungs, such isometries do exist. I perceived this to be a result which is very relevant to the OP; it in particular is an interaction between a combinatorial property of the abstract graph (number of rungs) and a concept studied in knot theory: slightly vaguely, one could say: Flapan gave a proof that evenfor rung-rung Möbius ladders are amphichiralnumbers $\geq 4$, whileeach odd-rung Möbius ladders areladder is non-amphichiral, while each even-rung Möbius ladder is amphichiral. The smallest example of the latter ladderladders is the embedding represented by the illustration
on p. 272 of loc. cit. about, when thought of to have precisely four rungs, about which Flapanloc. cit. says that it is non-is amphichiral, iwhile if the picture is thought of to represent a Möbius ladder with five rungs, then loc.e cit. says that there does not exist any orientation-reversing self-diffeomorphism ofdiffeomorphismof $\mathbb{R}^3$ which whouldwould fix it as a point set.
(The latter is a precise and usual statement of the matter. A more vague alternative statement one often encountered is 'It is not equivalent to its mirror-image.' wherein 'mirror-image' is either left undefined, or is defined via orientation-reversing self-homeomorphism of $\mathbb{R}^3$, whereupon the alternative statement becomes at least not simpler.)
The amphichirality of the even-rung Möbius ladders is easy to see, the substance of Flapan's results is non-amphichirality of the odd-rung Möbius ladders with at least 5 rungs.
The author on p. 272 of loc. cit. writes
which is a an example of what the OP is asking for: this meant to represent a non-knot embedded into $S^3$, namely the abstract undirected simple graph $M_3$, the three-rung Möbius ladder.
Furthermore, one should mention that there is a very recent preprint
Erica Flapan, Thomas Mattman, Blake Mellor, Ramin Naimi, Ryo Nikkuni: Recent Developments in Spatial Graph Theory. arXiv:1602.08122v2 [math.GT]
which is relevant to the OP. Therein, more results on embedded Möbius ladders are summarized, in particular the new paper (published after the OP)
E. Flapan and E. Davie Lawrence, Topological symmetry groups of Möbius ladders, J. Knot Theory Ramifications, vol. 23, no. 14, (2014)
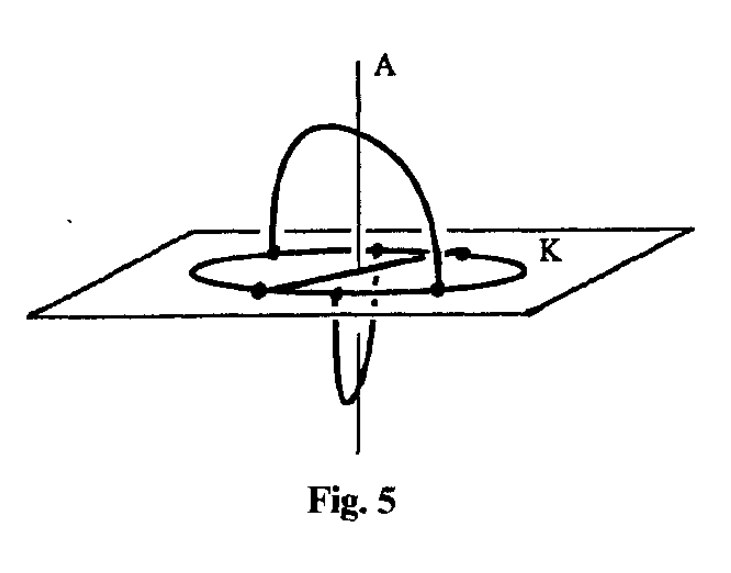
- I realized that there is an issue with the rendition I gave above: in loc. cit. the self-diffeomorphism of $S^3$ which is shown to be impossible is assumed to map what is called in loc.cit. the 'loop' $K$ of the geometrically Möbius ladder to itself, so strictly speaking, the above presentation claims that loc. cit. proved more than loc. cit. claims it proves. However, personally, I do not understand the hypothesis $h(K)=K$ in Theorem 2 of loc. cit. in the sense that to me it seems evident that the hypothesis $h(M_n)=M_n$ in Theorem 2 implies $h(K)=K$ (because of $h(M_n)=M_n$ $\Rightarrow$ the abstract graph-homomorphism $a$ defined by $h$ is a graph-automorphism of $M_n$ $\Rightarrow$ $a$ maps the abstract graph underlying the 'loop' $K$ to itself $\Rightarrow$ $h(K)=K$, the latter implication because $h$ is assumed to satisfy $h(M_n)=M_n$) so it seems it can simply be left out. This is what the footnote 1 is about.
1 Note that in loc. cit. there is a slightly stronger hypothesis than mere fixing the image of the embedding in its entirety. This hypothesis is essential in the special case $r=3$, yet can be left out for all $r\geq 4$, as loc. cit. itself points out on p. 272.
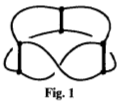
2 The rung-number $r=3$ is an exceptional case. The $3$-rung Möbius ladder is isomorphic to me, seems superfluousthe complete graph $K^{3,3}$, so I thinkand its geometric realization in $\mathbb{R}^3$ happens to be amphichiral: it is evident that the geometric graph $G$ represented by the illustration
in loc. cit. gives a proof of what is claimed in this threadisotopic to its 'mirror-image'.