This particular screensaver did not just nicely illustrate math, it actually motivated research:
A Tisket, a Tasket, an Apollonian Gasket, Dana Mackenzie
In the spring of 2007 I had the good fortune to spend a semester at the Mathematical Sciences Research Institute in Berkeley. Someone had installed a screen-saver program on the computer. Of course, it had to be mathematical. The program drew an endless assortment of fractals of varying shapes and ingenuity. Every couple minutes the screen would go blank and refresh itself with a completely different fractal. I have to confess that I spent a few idle minutes watching the fractals instead of writing.
One day, a new design popped up on the screen (see below). It was different from all the other fractals. It was made up of simple shapes—circles, in fact, and unlike all the other screen-savers, it had numbers! My attention was immediately drawn to the sequence of numbers running along the bottom edge: 1, 4, 9, 16 ... They were the perfect squares! Seeing those numbers awakened the math geek in me. What did they mean? And what did they have to do with the fractal on the screen? Quickly, before the screen-saver image vanished into the ether, I sketched it on my notepad, making a resolution to find out someday.
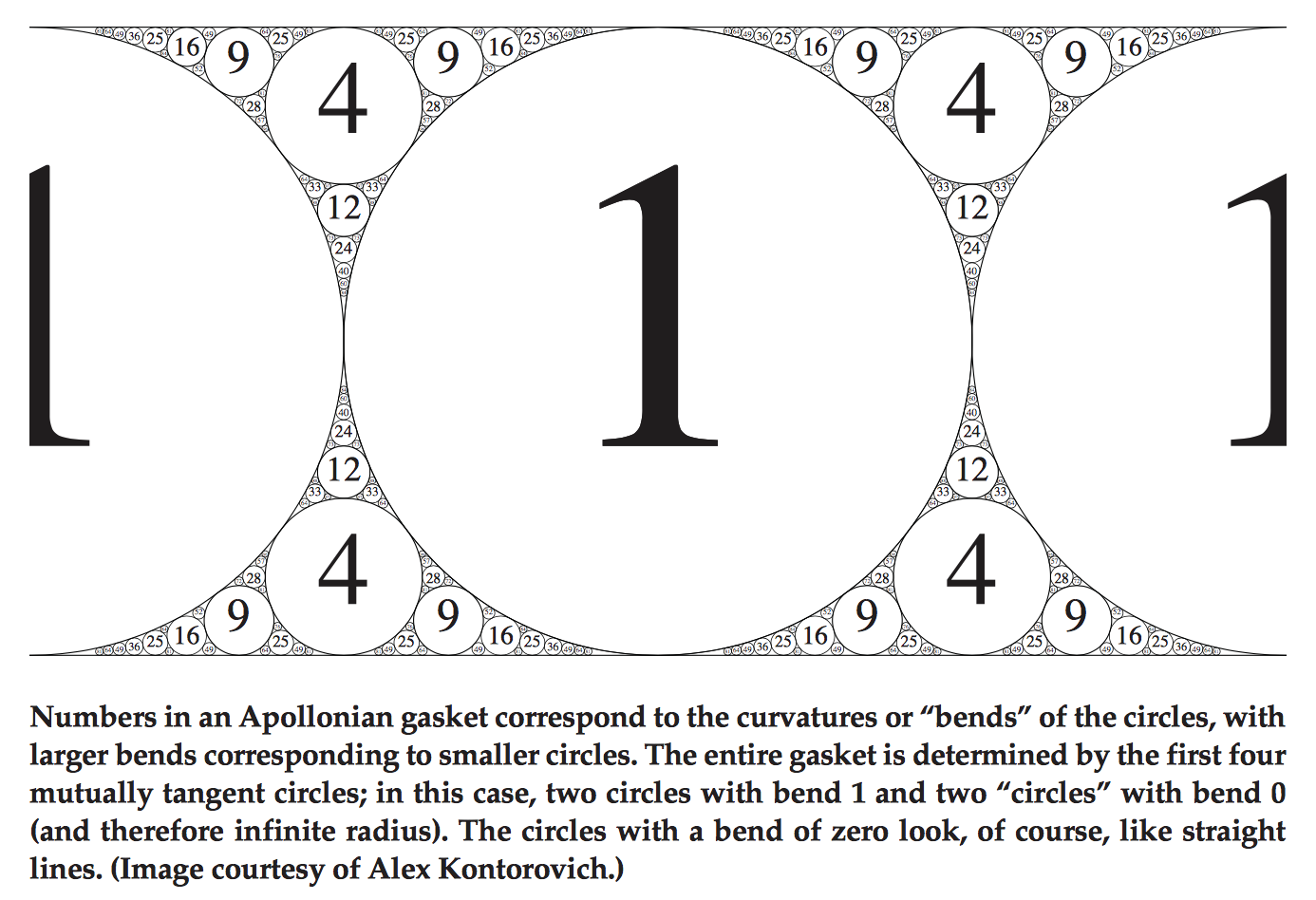
Here you can watch the screensaver in action, illustrating Descartes' theorem.
