Let $\alpha$ and $\beta$ be incommensurate real numbers.
Consider the function $f(x)={\rm cos}(x)+{\rm cos}(\alpha x)+{\rm cos}(\beta x)$ and its positive zeros $x_k(\alpha,\beta)$.
Fix $\alpha$ and $\beta$, and consider the distances between successive zeros $z_k=x_{k+1}-x_k$.
The concrete distribution of the zeros depends on $\alpha$ and $\beta$, but the distribution always shows singularities. Here are some examples for the first 100k zeros for various $\alpha$ and $\beta$:
distribution of zero distances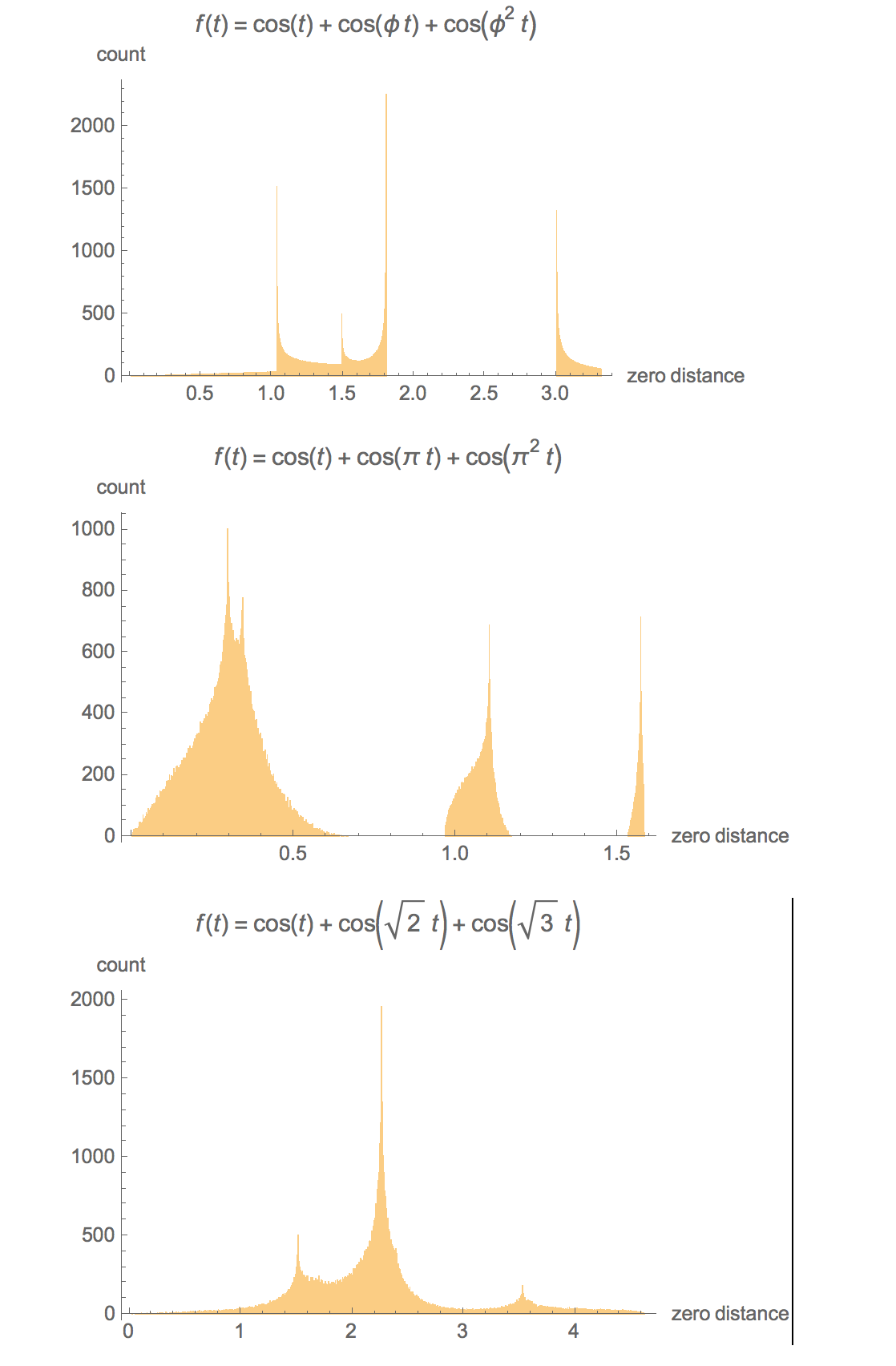
The position of the singularities does obviously depend on the continued fraction representation of $\alpha$ and $\beta$, as higher rational approximations to $\alpha$ and $\beta$ will display similar distributions.
My question is: Can one write down explicit approximations for the positions of these singularities in these distributions of the distances between the zeros in terms of the continued fraction expansions of $\alpha$ and $\beta$?
(The distances $z_k$ and $z_{k+1}$ are strongly correlated.)
The function $f(x)$ is almost periodic. In the literature about almost periodic functions I could not locate information about the distribution of the distances between the zeros.
Mathematica code to generate the images: https://drive.google.com/open?id=0B649LNvIOdYnaDctRElFYjQ2RlU
