For another example that homology and homotopy capture holes differently: note that the curve $C$ in the bitorus below is homologous to zero but not homotopic to zero.
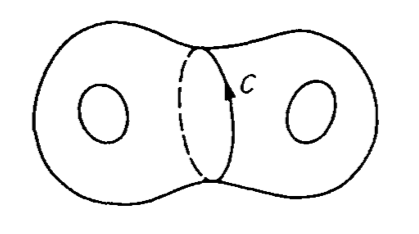
Also, a remarkable difference between them is that while an homology chain can be subdivided into smaller chains, a map from a sphere cannot be subdivided into smaller maps from a sphere. Historically, higher homotopy groups were only pursued after Hopf's fundamental example of a map $S^3 \to S^2$ not homotopic to zero, which was already commented above (I recommend the reading of H. Samelson, "$\pi_3(S^2)$, H. Hopf, W. K. Clifford, F. KleinKlein", In: History of Topology, p.575-578, Elsevier, Amsterdam, 1999.)
