One of applications of infinite Graph theory is about boiling points of infinite symmetric graphs in Nanotechnology .
Wiener showed that the Wiener index number is closely correlated with the boiling points of Alkane molecules see Wiener, H. J. "Structural Determination of Paraffin Boiling Points." J. Amer. Chem. Soc. 69, 17-20, 1947.
Let $G$ be a molecular graph with vertex set $V$. The Wiener index of $G$ which is denoted by $W(G)$ is defined by $\sum_{(u,v)\subset V\times V} d(u,v)$ where $d(u, v)$ denotes the distance between the vertices $u$ and $v$. Wiener index is topological invariant.
In fact Wienere index is invariant under the action of the automorphism group of the graph $G$. So the study of Wiener indices is correspond to study of topological invariant theory of graphs
For example for the case of Carbon Nanocone, which is infinite symmetric graph 
In fact Hyper-Wiener index is topological invariant and we calculated(when I was Bachelor degree) the hyper-Wiener index of the infinite one-pentagonal Carbon Nanocone. The graph of this molecule consists of one pentagon surrounded by layers of hexagons. If there are layers, then this graph is denoted by $G_n$
We showed the following explicit formula
$$WW(G_n)=20+\frac{533}{4}n+\frac{8501}{24}n^2+\frac{5795}{12}n^3+\frac{8575}{24}n^4+\frac{409}{3}n^5+21n^6$$
See
We also computed (when I was Bachelor degree)the Wiener index Dendrimer Nanostar which is infinite graph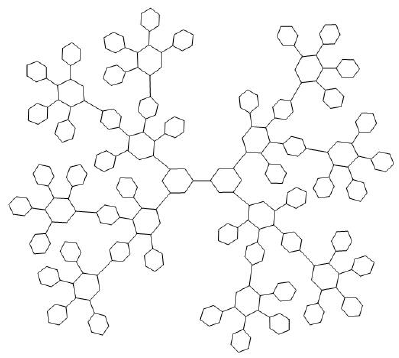
We showed the following explicit formula
$$W_{\{G_n\}} = 972n4^n−14584^n+17552^n−270$$
Moreover we computed(when I was bachelor degree) the Wiener index of a phenylenic pattern graph which is infinite symmetric graph.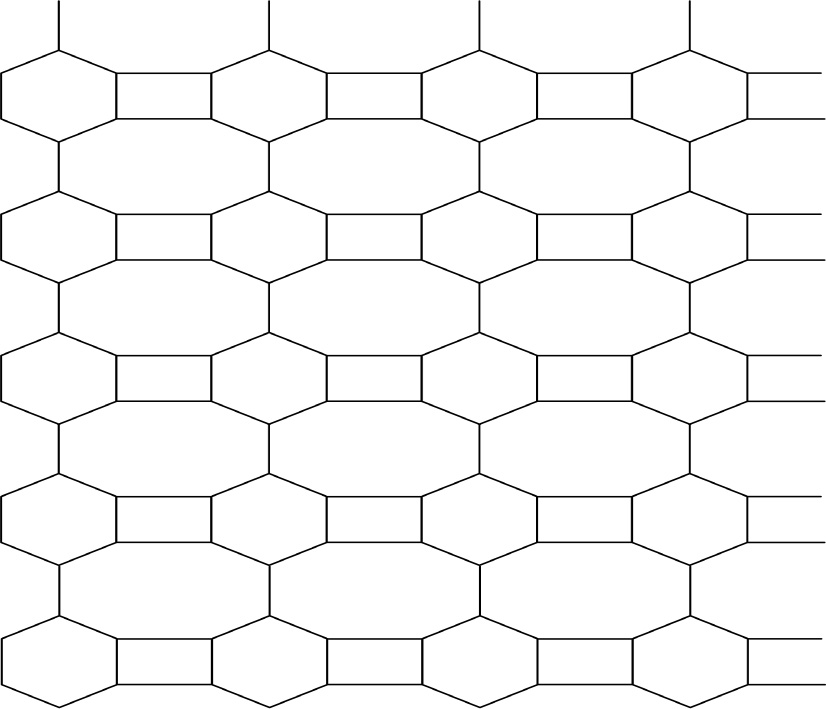
We showed that
$$W(G_{m,n})= − 4 − 64/5 n + 9m + 24m^3 + 30mn + 48m^3 n + 12m^2n + 24m^3n^2+ 18m^2n^2 − 18n^2 − 14n^3 + 6m^2n^3 + 39mn^2 − 6n^4 − 6/5 n^5 + 24n^3m + 6n^4m$$
See my Bachelor paper
