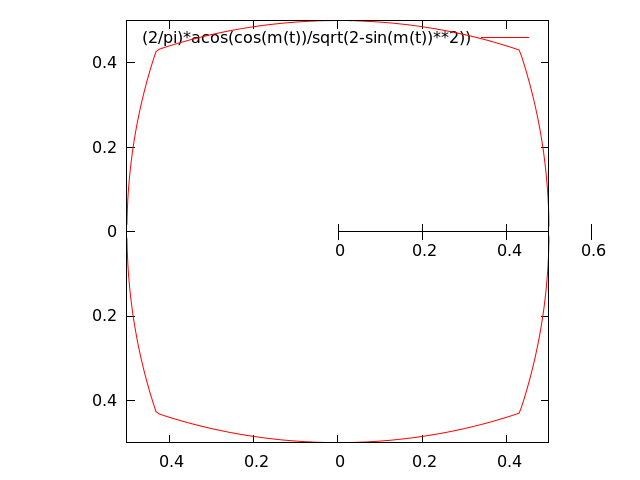
What follows is an illustration of $P$ for $d=2$ (the boundary of $P$ is in red, the boundary of $Q$ is, of course, the black square):
This map still needs to be modified a little: let $h\colon Q\to K$ be obtained by composing the nearest point projection $Q\to P$ (which makes sense since $P$ is convex compact) with the exponential map $P\to K$ (restricted to $P$, as explained above, where it is a homeomorphism). So $h$ is $1$-Lipschitz (and not injective on all of $Q$, although it is a homeomorphism on $P$). Also, $h$ commutes with all the symmetries of $(Q,K)$ because the construction is completely symmetric. In particular, the various copies of $h\colon Q_i\to K_i$ match at the boundaries, so we get a map $h\colon C\to S$ as announced.

