UPDATE
The integral in T. Amdeberhan's question was taken from his own paper that was written 10 years earlier before he posted his question: https://arxiv.org/abs/0808.2692 A dozen integrals: Russell-style. Thus it seems that T. Amdeberhan knew the answer to the question he asked. Why did he ask it then?
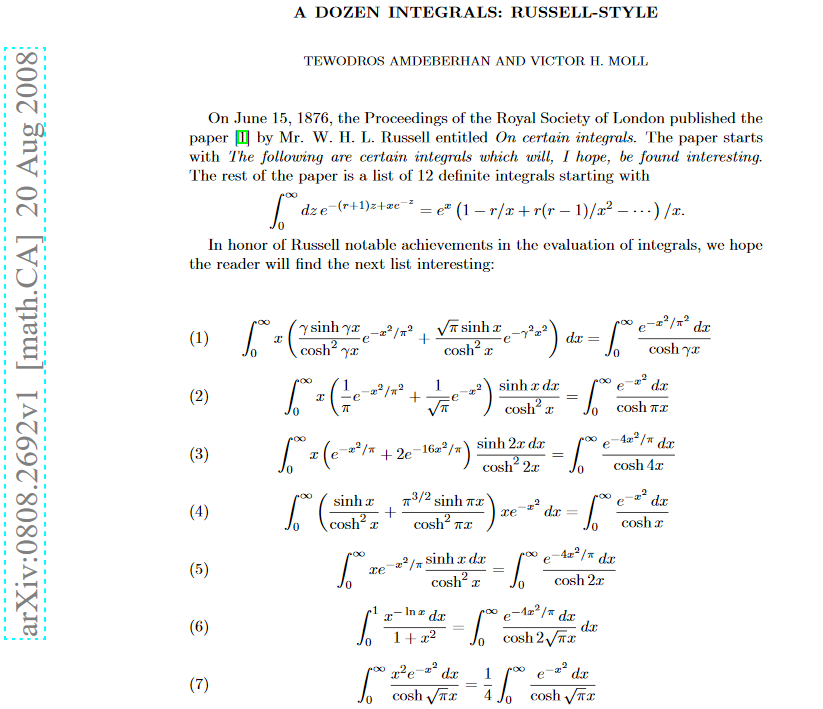
I provide a screenshot below for the reader's convenience (integral number $7$):
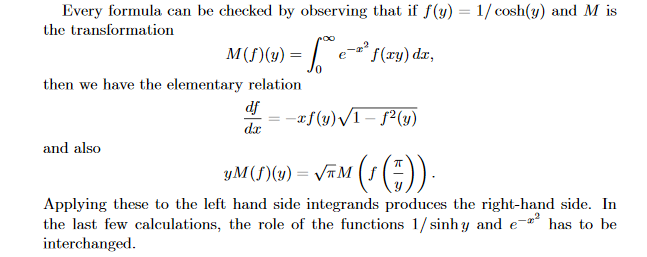
At the end of the paper, the authors provide a sketch of proof:
OLD ANSWER
Note that the following functions are self-reciprocal $\sqrt{\frac{2}{\pi}}\int_0^\infty f(x)\cos ax dx=f(a)$ (see this MO post for a list of such functions): $$ \frac{1}{\cosh\sqrt{\frac{\pi}{2}}x},\quad e^{-x^2/2}.\tag{1} $$ It was proved by Hardy (Quarterly Journal Of Pure And Applied Mathematics, Volume 35, Page 203, 1903) and Ramanujan (Ramanujan's Lost Notebook, part IV, chapter 18) that for two self-reciprocal functions $f$ and $g$ we have $$ \int_0^\infty f(x)g(\alpha x) dx=\frac{1}{\alpha}\int_0^\infty f(x)g(x/\alpha) dx. $$ The formal agument is as follows but it can be made rigorous for certain type of functions $$ \int_0^\infty f(x)g(\alpha x) dx=\int_0^\infty f(x)dx\cdot \sqrt{\frac{2}{\pi}}\int_0^\infty g(y) \cos(\alpha x y)dy=\\ \int_0^\infty g(y)dy \cdot \sqrt{\frac{2}{\pi}}\int_0^\infty f(x)\cos(\alpha x y)dx=\int_0^\infty g(y)f(\alpha y) dy=\\ \frac{1}{\alpha}\int_0^\infty f(x)g(x/\alpha) dx. $$ For functions in $(1)$ which decay rapidly at $x\to\pm\infty$ it is certainly true. So we obtain an identity due to Hardy and Ramanujan $$ \int_{0}^{\infty} \frac{e^{-x^{2}/2}}{\cosh{\sqrt{\frac{\pi}{2}}\alpha{x}}} {dx} = \frac{1}{\alpha} \int_{0}^{\infty} \frac{e^{-x^{2}/2}}{\cosh{\sqrt{\frac{\pi}{2}}\frac{x}{\alpha}}}{dx}. $$ After some simplifications it becomes $$ \int_{0}^{\infty} \frac{e^{-\alpha^2x^{2}}}{\cosh{\sqrt{{\pi}}{x}}} {dx} = \frac{1}{\alpha} \int_{0}^{\infty} \frac{e^{-x^{2}/\alpha^2}}{\cosh{\sqrt{{\pi}}{x}}}{dx}. $$ To complete the proof differentiate this with respect to $\alpha$ and then set $\alpha=1$.