Can $n$ circles on a plane generate $m$ intersection points where at least $k$ circles intersect?
For $k = 2$ the answer is obvious since we can always place circles so that every one of them intersects every other, generating in total at most $2 {n\choose{2}}$ intersection points of $2$ circles.
What can we say for $k = 3$? In particular I am interested in $n = 7$, $m = 12$.
It is known (see figure) that $8$ circles can generate $12$ intersection points of at least $3$ circles.
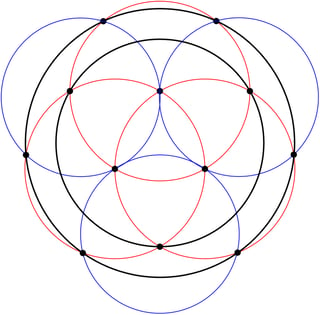
The question is if we: can we generate $12$ intersection points of at least $3$ circles using only $7$ circles in total?
