Although Emil JeřábekEmil Jeřábek is certainly right in that these, as Bjørn Kjos-HanssenBjørn Kjos-Hanssen called them, ultraupsets, seem to be quite complicated, I would like to propose a combinatoric-topological reformulation which I think makes them more "touchable".
If we switch to complements, we are looking at abstract simplicial complexes with a very special property - they contain exactly one from each pair of complementary simplices.
It seems that some of the consequences can be more easily understood with the aid of this geometric intuition. In particular one can visualize such complexes in low dimensions.
Among subcomplexes of an 1-simplex, only single points are possible.
For subcomplexes of a triangle, there are two possibilities: an edge of the triangle, and the discrete 3-element set of its vertices.
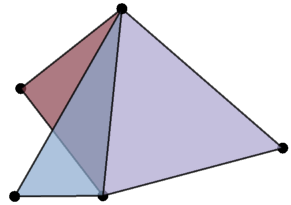
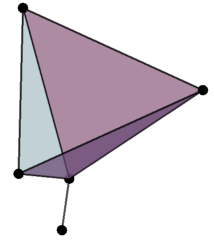
For a tetrahedron, one has three (up to isomorphism) possibilities: a 2-face, the disjoint union $($boundary of a triangle$)\cup($point$)$, and three edges meeting at a vertex.
For a 4-simplex one gets:
- a 3-face;
- disjoint union $($boundary of a tetrahedron$)\cup($point$)$;
- two kinds of complexes with 7 edges:
and
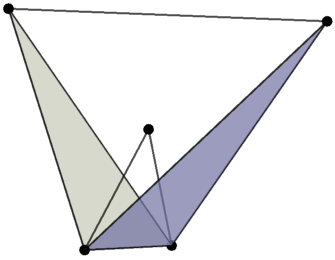
- a complex with 8 edges
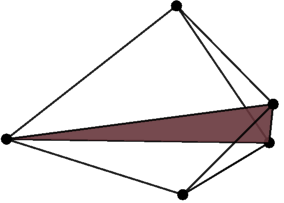
- one with 9 edges
- and the whole 1-skeleton.
All in all this seems to be a very interesting combinatorial object.