Erdős conjectured that, except $1, 4$ and $256$, no power of $2$ is a sum of distinct powers of $3$.
A vice-versa conjecture may be: except $1$, $9$ and $81$, all powers of $3$ contains two consecutive powers of $2$ or/and two missed consecutive powers of $2$ in their binary expansions.
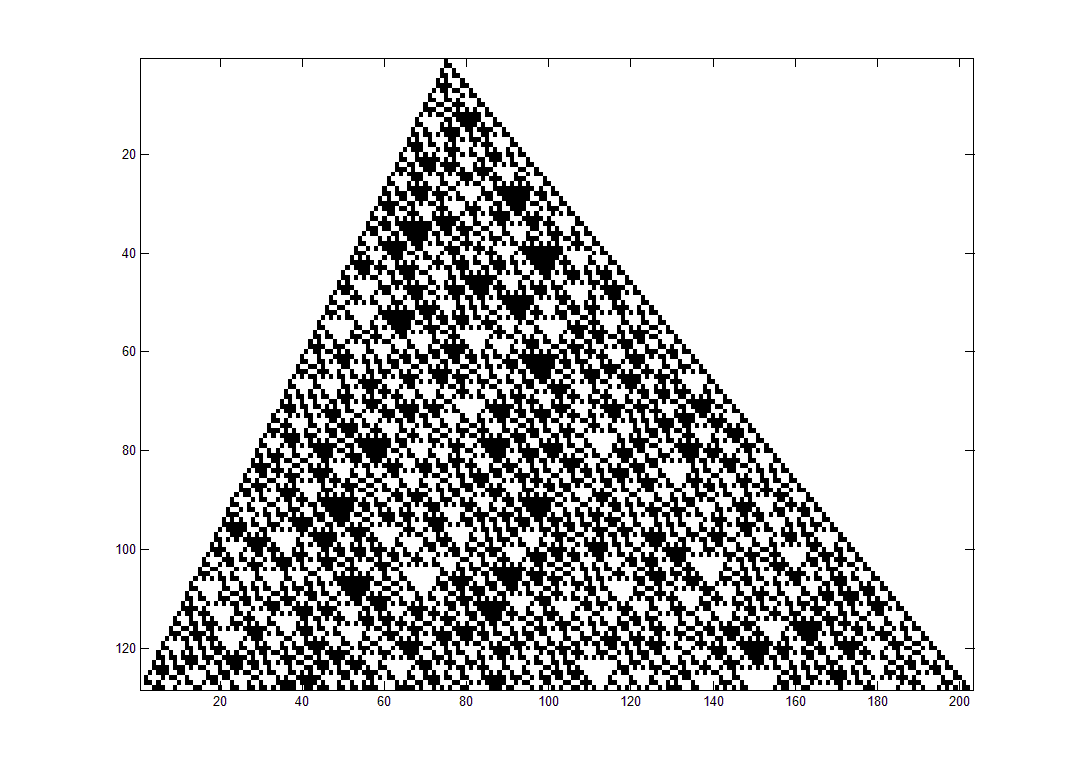
We can generate the powers $(3^n)$ as a cellular automaton where the $n$-th line represents the binary expansion of $3^n$ and we can observe that the conjecture is still verified as showed in the next image with 128 lines.