there is something called the Altland Zirnbauer classification of topological insulators which is related to both the random matrices and Bott periodicity
https://golem.ph.utexas.edu/category/2014/07/the_tenfold_way.html
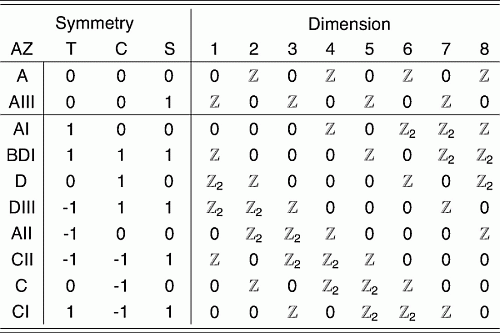
Novel Symmetry Classes in Mesoscopic Normal-Superconducting Hybrid Structures (Altland + Zirnbauer)
Periodic table for topological insulators and superconductors (Kitaev)
Attempt at Discussion
As typical in QFT there is a difficult Hamiltonian (this is Hartree-Fock approximation of some electric field):
$$ \hat{H} = \int d^d x \; \Bigg( \sum_{\sigma, \tau \in \uparrow ,\downarrow} \psi^\dagger_\sigma h_{\sigma \tau}\psi_\tau + \Delta \psi^\dagger_\uparrow \psi^\dagger_\downarrow + \Delta^\ast \psi_\uparrow \psi_\downarrow \Bigg)$$
for reasons one might challenge this can be written in terms of creation an anihilation operators:
$$ \hat{H} = \sum_{\alpha \beta} \big( h_{\alpha\beta} c^\dagger_\alpha c_\beta + \frac{1}{2} \Delta_{\alpha\beta} c_\alpha^\dagger c_\beta^\dagger + \frac{1}{2} \Delta_{\alpha\beta}^\ast c_\alpha c_\beta \big) $$
The indices instead of ranging from 1 thru N, both indices $\alpha, \beta$ range over the sites of a lattice. This Hamiltonian is tell us what happens at the edges.
In layman's terms: it's too complicated! So we will approximate with random matrix ensemble.
$$ \hat{H} = \frac{1}{2} ( \begin{array}{cc} \mathbf{c}^\dagger & \mathbf{c} \end{array} ) \left( \begin{array}{cc} h & \Delta \\ -\Delta^\ast & h \end{array} \right) \left( \begin{array}{c} \mathbf{c}^\dagger \\ \mathbf{c} \end{array} \right) \equiv \frac{1}{2} ( \begin{array}{cc} \mathbf{c}^\dagger & \mathbf{c} \end{array} ) \; \mathcal{H} \;\left( \begin{array}{c} \mathbf{c}^\dagger \\ \mathbf{c} \end{array} \right) $$
This is how Altland+Zirnbauer define the "Bogliubov-de Gennes Hamiltonian" $\mathcal{H}$ which acts on the space of spins at all points $\mathbf{C}^{2N} \times \mathbb{C}^2$.
Then we can examine each symmetry class (to be continuedA, AIII, AI, D, C, CI, etc) and check for various types of time-reversal or spin-rotation or charge-conjugation. And he will obtain various generalized GUE/GOE/GSE etc.
(to be continued...)
K-theory
Kitaev seems to feel the Altland-Zirnbauer classification was incomplete in dimensions > 3. He is also the first to mention Bott periodicity.
Here K-theory is read literally as "linear algebra over matrices with elements that are functions of the base space". Classical random matrix theory is a 0-dimensional case where the base space is $\{ pt \} $. In higher dimensions these become related to the Clifford algebras.
(More to say as I learn.)
