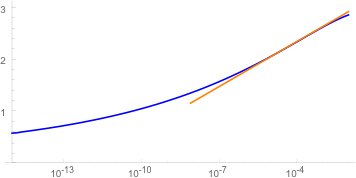
the small-$\rho$ asymptotics of $$I(\rho)=-\int_{1.1}^{\infty}\frac{\sin(k\rho)}{\rho k^{1.9}\ln k}\,dk$$
is governed by the large-$k$ behavior of the integrand, which gives $I(\rho)\propto \rho^{1.9-2}$ up to a logarithmic factor, and a numerical evaluation supports the asymptotic
$$I_{\rm asymp}(\rho)=-\rho^{-0.1}(3.5+0.126\ln\rho)$$
http://ilorentz.org/beenakker/MO/loglinearplot.png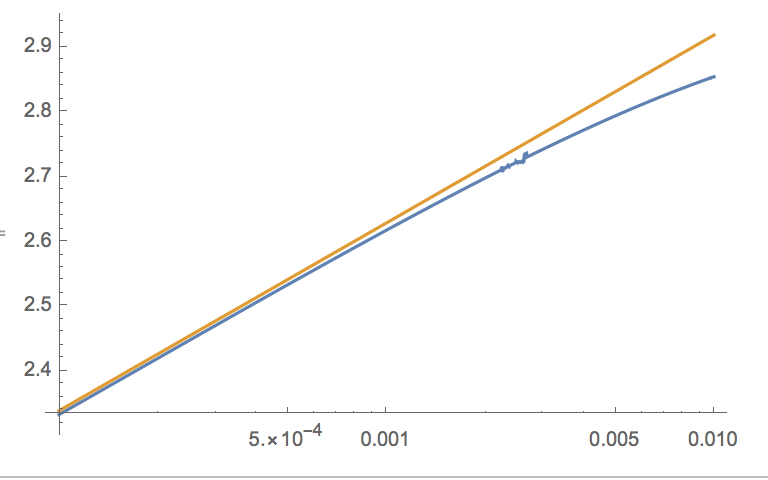
Log-linear plot of $-\rho^{0.1}I(\rho)$ (blue line) and $-\rho^{0.1}I_{\rm asymp}(\rho)$ (orange line)
UPDATE, following Bazin's recent answer:
I have some convergence issues with a numerical evaluation at much smaller $\rho$, but if I can trust the Mathematica output the curve does seem to level off towards an asymptote at zero, as derived by Bazin, possibly as $I(\rho)=-\text{constant}\times(\rho^{0.1}\log\rho)^{-1}$.
http://ilorentz.org/beenakker/MO/asymptote_2.png