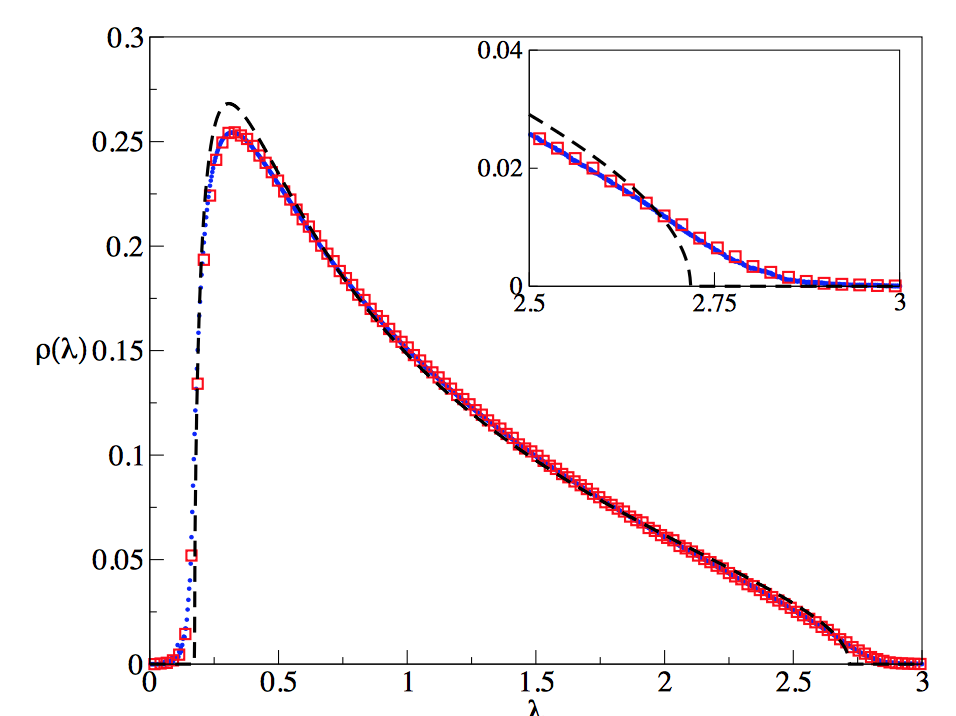
A general theoretical framework has been developed in Cavity Approach to the Spectral Density of Sparse Symmetric Random Matrices (2008), and an alternative approach is in Spectral Density of Sparse Sample Covariance Matrices (2006). Here is a plot for a binary $\pm 1$, rather than Gaussian, distribution of the nonzero entries ($N=4000$, $\alpha=M/N=0.3$, $p=d/N=12/4000$). The red points are a numerical simulation, the blue points are the "cavity theory" from the 2008 paper and the dashed curve is the "effective medium theory" from the 2006 paper. As you can see, both theories are of comparable quality, except near the spectral edge, where the former is better.
http://ilorentz.org/beenakker/MO/sparse_matrices.png