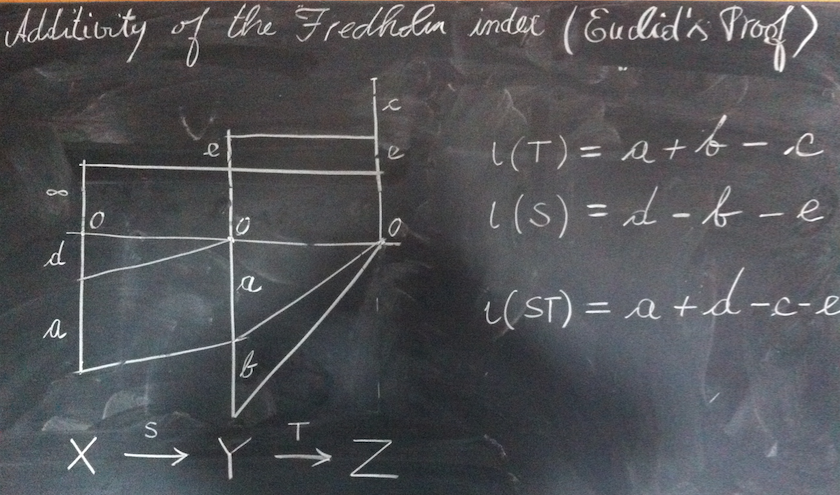
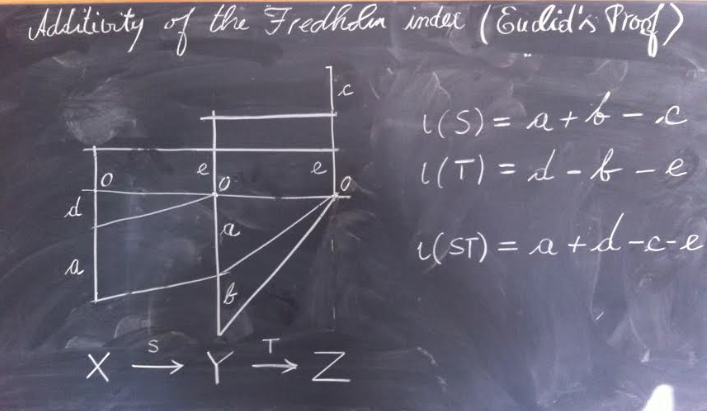
I was somehow challenged by the idea: do more abstract topics allow proofs without words? I came out with this example. Of course, it is disputable if it is really "without words", since some words of explanation should be given (here they are: vertical segments represent Banach spaces and subspaces; connecting segments between two of them represent a linear operator. Italic letters $a,b,c,d,e$ are the dimensions of the corresponding linear subspaces ).