Yes, there do exist sufficient conditions for asymptotic stability when the Lyapunov function is negative semi-definite, which I describe below.
Krasovsky's Theorem
Given an autonomous ODE $\dot x = f(x)$ with fixed point at the origin. Let $K$ be a manifold that does not contain entire trajectories of the ODE. If there exists a Lyapunov function $V$ such that the orbital derivative $\dot V$ satisfies:
- $\dot V<0$ outside of $K$
- $\dot V=0$ on $K$
then the dynamics is asymptotically stable.
If $K = \{ x ~:~ F(x)=0\}$, here is a sufficient condition for $K$ to not contain entire trajectories of the ODE: $$ (f^T \nabla F)(x) \ne 0 \quad \text{on $K \setminus \{ \mathbf{0} \}$} $$ where $f$ is the vector field of the ODE. (This just ensures that the vector field $f$ is never orthogonal to the normal to the surface.)
Example
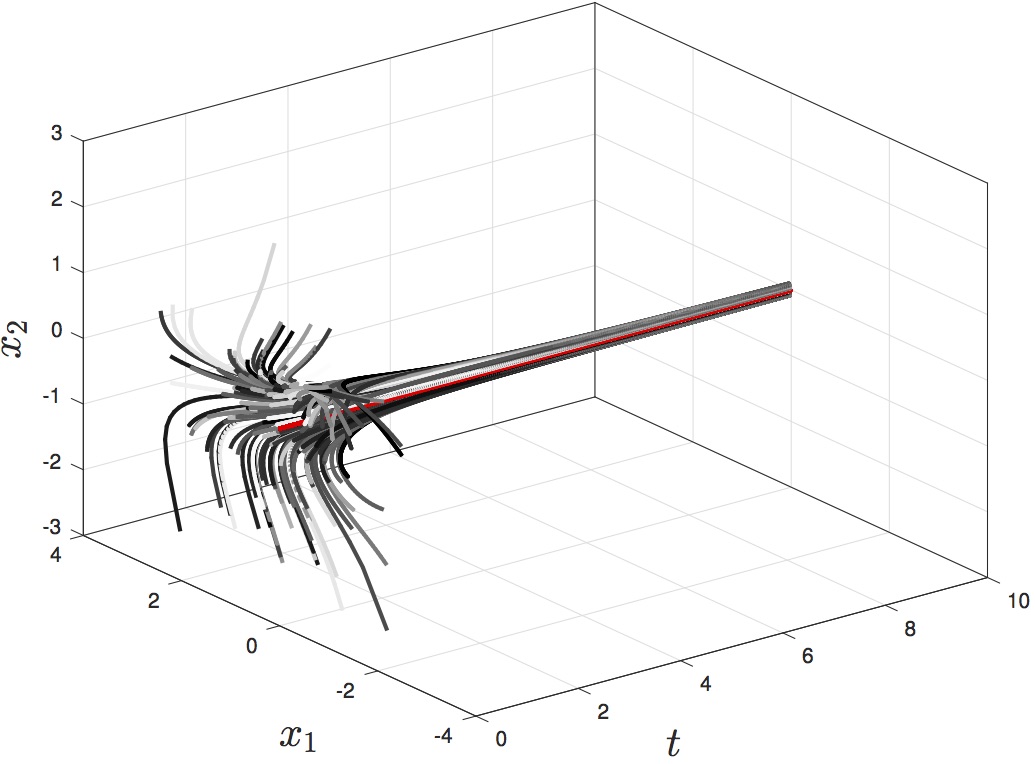
Consider the ODE: $ \dot x_1 = -x_1 + 3 x_2^2 \;, \dot x_2 = - x_1 x_2 - x_2^3 \;. $ In this case, a Lyapunov function is given by $V(x_1,x_2)=(x_1^2+x_2^2)/2$, whose orbital derivative is negative semi-definite. The set where $\dot V=0$ is given by the zero level set of the function $F(x_1,x_2)=x_1 - x_2^2$. Note that $(f^T \nabla F)(x_1,x_2) = 2 x_2^2 + 4 x_2^4 \ne 0$ on $K \setminus \{ \mathbf{0} \}$. Thus, by Krasovsky's Theorem the origin is asymptotically stable as illustrated in the graphic below.
In this graphic, two of the axes correspond to state variables $x_1$ and $x_2$, and the other axis is the time variable $t$. The red line marks the state $x_1=0$ and $x_2=0$ for the time interval shown. Different grey shading is used for trajectories with different initial conditions.
ADD
Here is a cartoon from the book referenced below, which illustrates the idea behind Krasovsky's Theorem. The dark line labelled $\gamma$ represents a solution of the ODE, the lighter lines are contour lines of the semi-definite Lyapunov function $V$, and the dashed region is $K$ where $\dot V=0$.
 This cartoon nicely illustrates how the dynamic avoids getting stuck inside $K$, and instead, asymptotically reaches a fixed point. Note that if the ODE solution enters $K$ then the value of the Lyapunov function does not change. This is illustrated by the curve remaining on a level curve of $V$ in the dashed region labelled $K$. However, eventually the ODE solution must exit $K$ (by hypothesis of Krasovky's Theorem), after which the Lyapunov function again decreases.
This cartoon nicely illustrates how the dynamic avoids getting stuck inside $K$, and instead, asymptotically reaches a fixed point. Note that if the ODE solution enters $K$ then the value of the Lyapunov function does not change. This is illustrated by the curve remaining on a level curve of $V$ in the dashed region labelled $K$. However, eventually the ODE solution must exit $K$ (by hypothesis of Krasovky's Theorem), after which the Lyapunov function again decreases.
This picture suggests that a set of non-isolated fixed points can be reached in this fashion.
Reference
David R. Merkin [1997]. Introduction to the Theory of Stability. Texts in Applied Mathematics. Springer. Translated from Russian by Andrei L. Smirnov and Fred Afagh.