Comments to the question contain several contributions to alternative approaches by Uri BaderUri Bader. Since the latter seems to be reluctant to collect them into an answer, I decided to do the part I understand.
Approach 1.
Suppose given $P$ with top and bottom; then removing top and bottom from $P\times\{1,...,n\}$ is the union of $B:=(P\setminus\{\text{bottom}\})\times\{1\}$, $M:=P\times\{2,...,n-1\}$ and $T:=(P\setminus\{\text{top}\})\times\{n\}$. The hypothesis is that $\{2,...,n-1\}$ is not empty, so all three are obviously contractible, $B$ attached to $M$ along $(P\setminus\{\text{bottom}\})\times\{1,2\}$ and $M$ to $T$ along $(P\setminus\{\text{top}\})\times\{n-1,n\}$. If I understand Uri Bader's comment correctly, one just contracts $B$ and $T$ to $M$ separately.
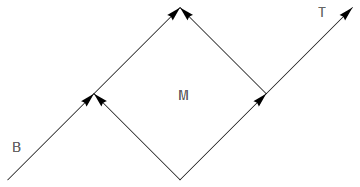
Sort of an illustration, with $P=\{\text{top},\text{bottom}\}$ and $n=4$:
Another one, with $P=2\times2\times2$ and $n=3$:
Approach 2.
This I turn upside down since I am more used to it. For any element $a$ of a (bounded (finite)) lattice $L$ the embedding $[\text{bottom},a]\hookrightarrow L$ has a right adjoint $a\land\_$. Call an element $d$ of a lattice $L$ $\textit{dense}$ if $\forall x\in L\ (d\land x=\text{bottom})\Rightarrow(x=\text{bottom})$ holds. For any nontrivial ($\ne\text{top}$) such element the restriction of the adjoint $d\land\_$ to $L\setminus\{\text{top},\text{bottom}\}$ lands on $(\text{bottom},d]$, so the latter (which has top $d$, hence is contractible) becomes a deformation retract of $L\setminus\{\text{top},\text{bottom}\}$.
Now invoking the comment by Richard Stanley - in case $L$ is distributive, it has no nontrivial dense elements if and only if it is a Boolean algebra.
A natural question here is whether a characterization is known of those general (non-distributive) lattices which do not possess nontrivial dense (neither codense) elements.
Note that for non-distributive lattices generality of this approach is somehow orthogonal to that in Tom Goodwillie's answer: the latter works for products of not necessarily lattices having tops and bottoms, while here one approaches lattices which might not decompose into products.
In this connection there was a very interesting comment by Dan Petersen which has been elucidated by Uri Bader, but unfortunately I do not know this area well enough to say anything definite. The way I understand the idea is to consider homotopy types arising from lattices constructed in the same way in different characteristics as sort of "$q$-analogues", that is, families of homotopy types depending on a "modular" parameter encoded in $q$. I don't really know what that means that I wrote.
Finally, - it might be true that face lattices of polytopes with top and bottom removed actually have the same homotopy types as the polytopes themselves. Does anybody know anything about it? Just a couple of considerations: if this is the case then obviously the answer to my second question is that any homotopy type of a finite CW-complex may occur. Indeed if I am not mistaken already simplices of the second barycentric subdivision of any CW-complex form a (topless) lattice. Note also that for a poset one possible version of the barycentric subdivision is formed by linearly ordered subposets, and maximal such are not altered if one removes top and bottom...