K.S. Kölbig, On the zeros of the incomplete gamma functions. This paper is from 1972, it has a whole list of references to older literature.
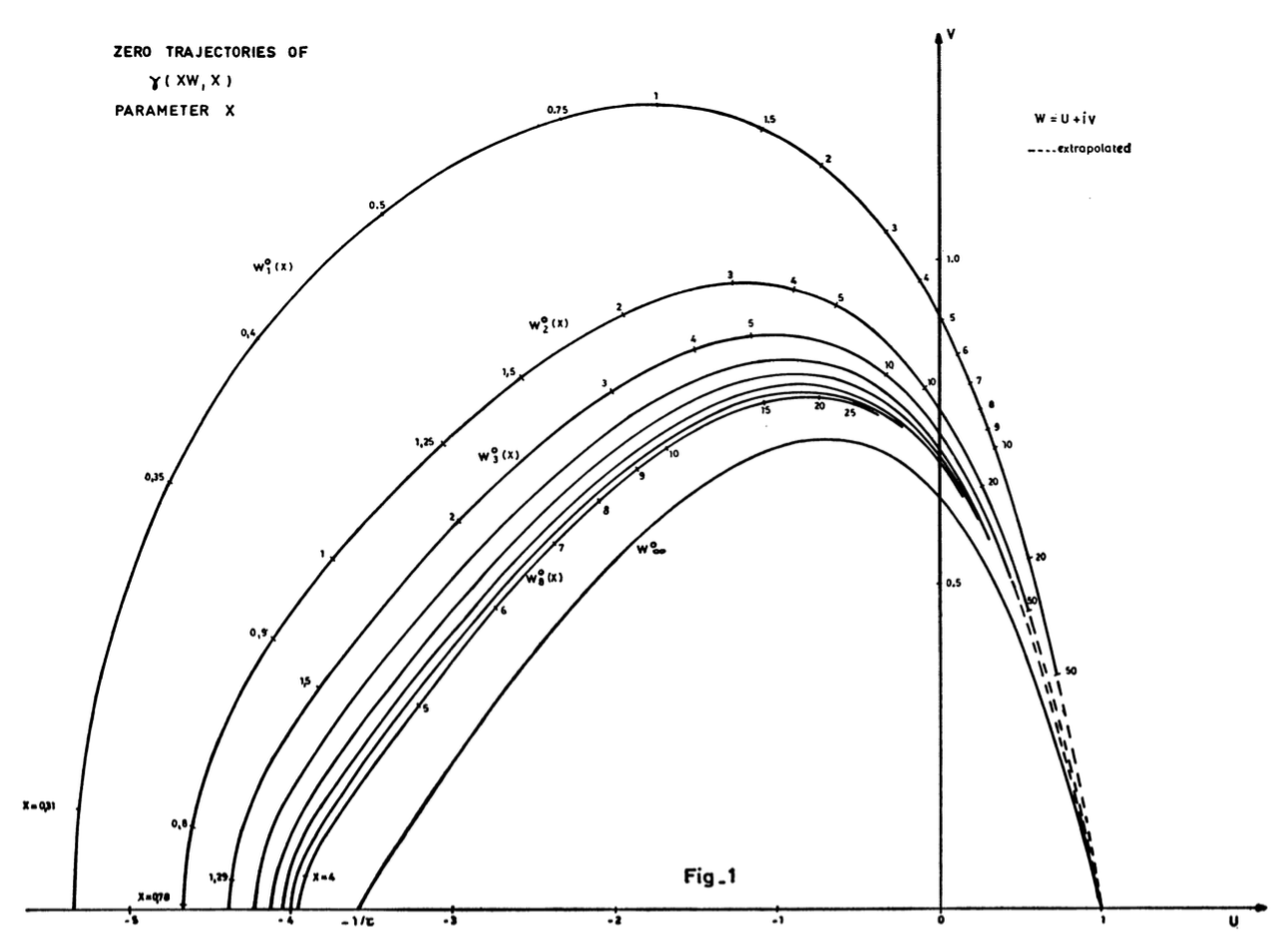
It is the aim of this note to recall some of the earlier results (occasionally correcting them), and to present a plot containing a few of the zero trajectories of the function $\gamma(xw, x)$ in the complex $w$-plane ($w = u + iv$), as functions of the real parameter $x$ > 0. It will be seen that these trajectories all lie in a finite region of the $w$-plane, and that they cluster towards a limiting curve as shown by Mahler (1930).
This limiting curve is interesting, it is given by $${\rm Re}\,(w\log w-w+1)=0.$$ In the plot below (from Kölbig's paper), you can see how the zeroes approach the limiting curve.
http://ilorentz.org/beenakker/MO/Kolbig.png