Well, yes, but no. So the function obtained by removing from the usual $L$-series of $E$ all the supersingular factors is probably not a very nice function. I doubt that it has an analytic continuation and hence I am not sure we should talk about its order of vanishing at $s=1$. But I do not know enough about these things to make that more precise, maybe someone else will correct me on this.
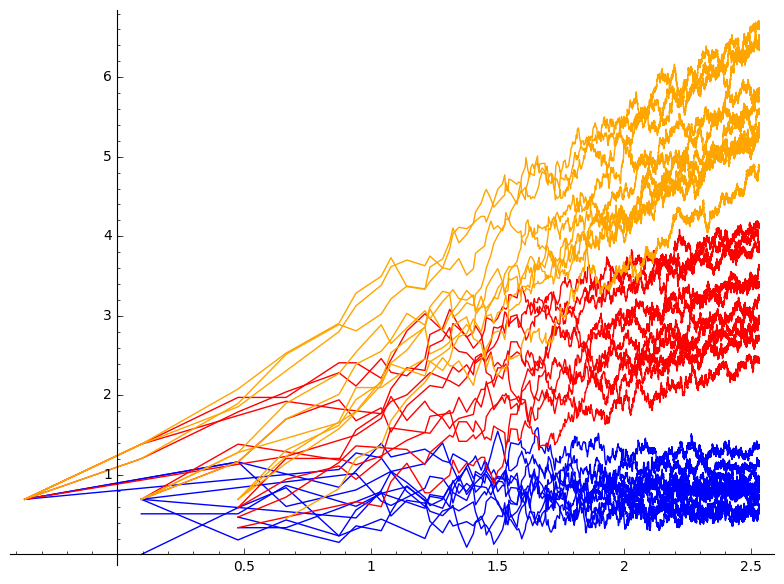
On the other hand, the original idea of looking at the product of $N_p/p$ where $N_p$ is the number of solutions modulo $p$ still makes sense. Let $f(x) = \log(\prod_{p\leq x} N_p/p)$, the earliest version of the conjecture of Birch and Swinnerton-Dyer says that $f(x)$ grows like $r\log\log(x)$ where $r$ is the rank. The plot below is $f(x)$ against $\log\log(x)$. I took the first ten curves of rank $0$ (blue) $1$ (red) and $2$ (orange) in Cremona's tables, but now running only over prime $p\leq x$ that are good ordinary. So it looks very much like this conjecture does not care about the few supersingular primes - without much surprise.