It looks to me like $D$ is not bounded. I have produced an animation using Maple. For $a$ running from $1$ to $40$ we take $b = 5a$, and plot the curves $\text{Re}(f(x+iy))=0$ (blue) and $\text{Im}(f(x+iy))=0$ (red) for $0 < x,y < 1/2$. The intersections of red and blue curves are off-axis zeros of $f$. It certainly appears that there are such zeros, and thus that the ray $b=5a$, $a > 1$, is contained in $D$. I suspect that it is possible to prove this using the Argument Principle.

It looks to me like $D$ is not bounded. I have produced an animation using Maple. For $a$ running from $1$ to $40$ we take $b = 5a$, and plot the curves $\text{Re}(f(x+iy))=0$ (blue) and $\text{Im}(f(x+iy))=0$ (red) for $0 < x,y < 1/2$. The intersections of red and blue curves are off-axis zeros of $f$. It certainly appears that there are such zeros, and thus that the ray $b=5a$, $a > 1$, is contained in $D$. I suspect that it is possible to prove this using the Argument Principle.

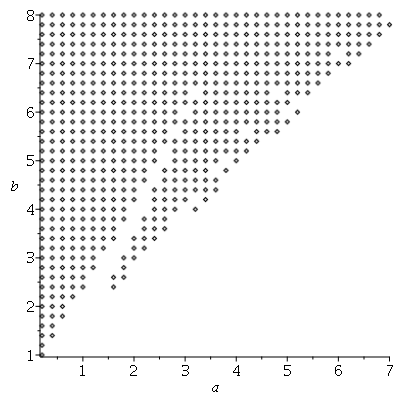
EDIT: For various points in the first quadrant of the $(a,b)$ plane, I computed the number of zeros in the rectangle $0.01 \le \text{Re}(z) \le 2, 0.01 \le \text{Im}(z) \le 2$, by numerically evaluating the integral of $(2 \pi i)^{-1} f'(z)/f(z)$ around the boundary of this region. Here is the result, showing the points for which there is at least one such zero. There may be some tantalizing hints of structure here. What seems to happen is that off-axis solutions bifurcate from zeros of multiplicity $2$ on the imaginary axis at certain $(a,b)$ values.