I will add two examples of conjectures from quantum topology based on computer data.
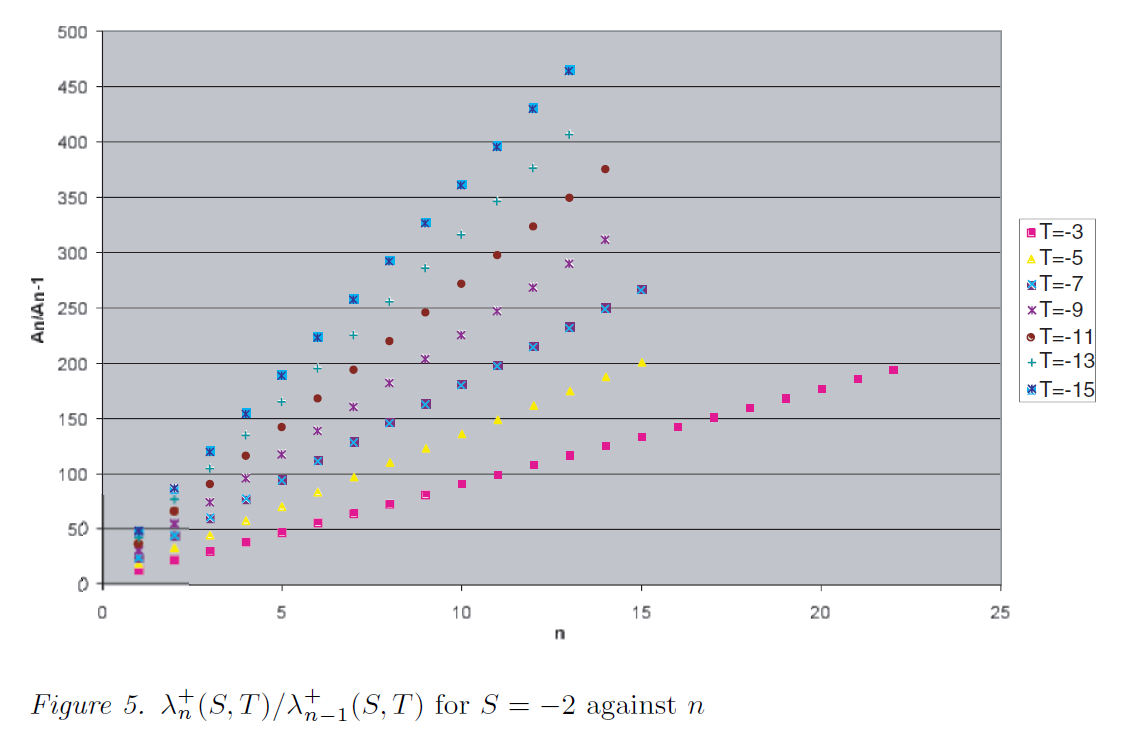
- The slope conjecture: Quantum topology associates a sequence of rational numbers to a rational homology sphere $M$ as coefficients of an invariant called the Ohtsuki series. The slope conjecture states that the ratios of adjacent coefficients of powers $(q+1)^n$ in the Ohtsuki series of a rational homology sphere (except $S^3$) asymptotically grows linearly in $n$ and that the slope satisfies certain conditions. I believe it is still wide open. It was discovered by Lawrence and Jacoby in this paper by examining compuercomputer-generated plots such as the following:
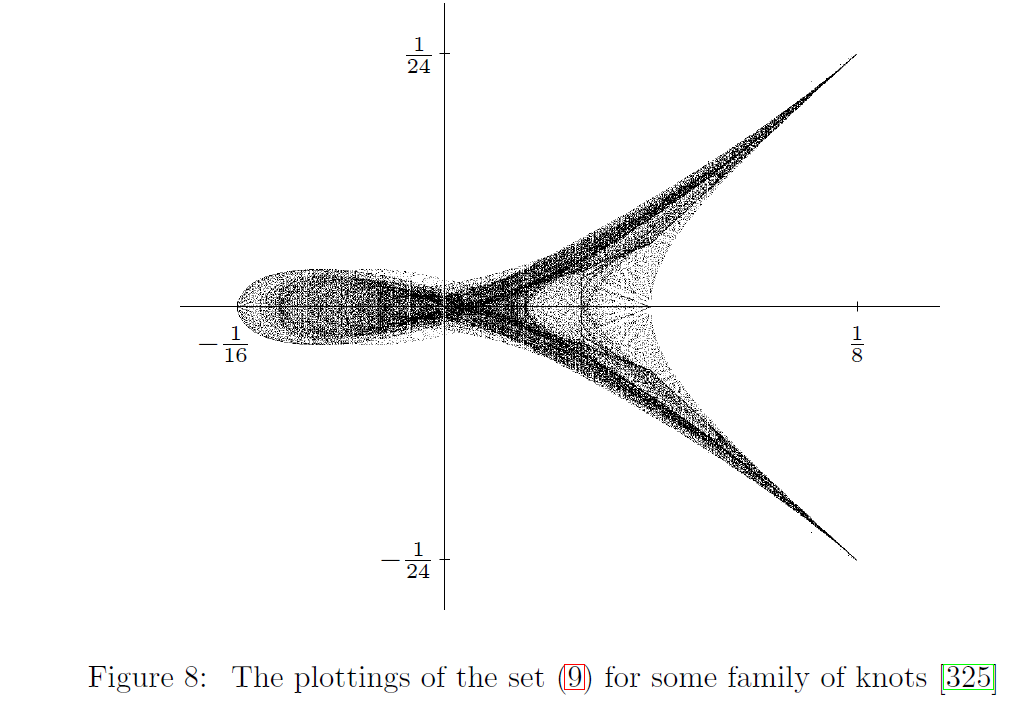
- Mysterious fish-like graph: Let $v_2$ and $v_3$ denote the $\mathbb{R}$-valued Vassiliev invariants of knots of degree $2$ and $3$ respectively, normalized appropriately. Willerton, following Okuda, plotted the values of $\left(\frac{v_2}{n^2},\frac{v_3}{n^3}\right)$ for certain families of knots with fixed crossing number, and obtained the following mysterious "fish-like graph":
This led to a slew of results and conjectures, as described in Ohtsuki's problem list. To the best of my knowledge, the although there are partial results, the appearance of the fish-like graph is still a mystery.