As pointed out in Piotr Achinger's answer, what follows is just an attempt to solve Exercise IV.2 1(c) (p. 260) in Gelfand & Manin.
Trying to use the above comments by Dylan Wilson, seems like $V_p$ is the convex hull of edge midpoints of $\Delta^{p+1}$.
Its facets are $p+2$ copies of $V_{p-1}$ (convex hulls of edge midpoints of each of the $p+2$ facets of $\Delta^{p+1}$) and $p+2$ copies of $\Delta^p$ (obtained at each of the $p+2$ vertices of $\Delta^{p+1}$ as a result of truncation (of length up to edge midpoints, which is the harshest possible truncation until truncating hyperplanes begin to intersect inside the polyhedron)).
In addition to the facets described in two bullets at the end of the question, there is one more copy of $V_{p-1}$ corresponding to the composable $(p-1)$-tuple $\operatorname{cone}(f_1)\to\operatorname{cone}(f_2f_1)\to\operatorname{cone}(f_p\cdots f_1)$, and$$\operatorname{cone}(f_1)\to\operatorname{cone}(f_2f_1)\to...\to\operatorname{cone}(f_p\cdots f_1),$$and $p+1$ more copies of $\Delta^p$ corresponding to composable $p$-tuples \begin{align*} &\operatorname{cone}(f_1)\to\operatorname{cone}(f_2f_1)\to...\qquad \to\operatorname{cone}(f_{p-1}\cdots f_1)\to\operatorname{cone}(f_p\cdots f_1)\xrightarrow*X_0,\\ &\operatorname{cone}(f_2)\to\operatorname{cone}(f_3f_2)\to...\qquad\qquad\qquad \to\operatorname{cone}(f_p\cdots f_2)\xrightarrow*X_1\to\operatorname{cone}(f_1),\\ &\operatorname{cone}(f_3)\to\operatorname{cone}(f_4f_3)\to...\qquad\qquad\qquad\ \quad \xrightarrow*X_2\to\operatorname{cone}(f_2f_1)\to\operatorname{cone}(f_2),\\ &\vdots\\ &\operatorname{cone}(f_i)\to...\to\operatorname{cone}(f_p\cdots f_i)\xrightarrow*X_{i-1}\to\operatorname{cone}(f_{i-1}\cdots f_1)\to...\to\operatorname{cone}(f_{i-1}),\\ &\vdots\\ &\operatorname{cone}(f_p)\xrightarrow*X_{p-1}\to...\ \to\operatorname{cone}(f_{p-1}f_{p-2}f_{p-3})\to\operatorname{cone}(f_{p-1}f_{p-2})\to\operatorname{cone}(f_{p-1}),\\ &X_p\to\operatorname{cone}(f_p\cdots f_1)\to...\ \quad\to\operatorname{cone}(f_pf_{p-1}f_{p-2})\to\operatorname{cone}(f_pf_{p-1})\to\operatorname{cone}(f_p), \end{align*} where maps with stars denote maps with degree shift by one; that is, $A\xrightarrow*B$ means a map of the form $A\to\Sigma B$ (or else $A\to B[1]$, or $\Omega A\to B$, or...).
Hope the maps and the patterns are clear from the above. If not, tell me, I'll try to formulate more details.
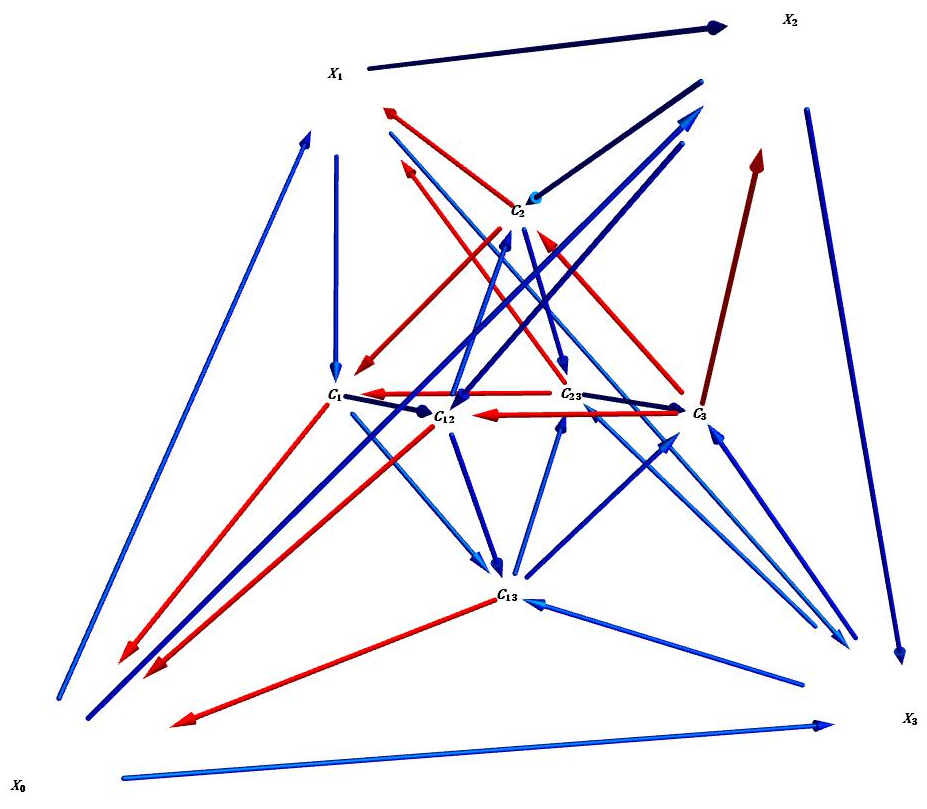
Here is a projection of $V_3$ to the 3-space ($C_{ij}$ stands for $\operatorname{cone}(f_j\cdots f_i)$; red means degree 1)
One can see it inside out on Wikipedia under the name of rectified 5-cell