(EDIT. Images have been added below.)

As far as I understand, any non-rectifiable curve is not chord arc. So any such curve will do, e.g. the Julia set of $z^2+c$ for $c\neq 0$ in the main cardioid of the Mandelbrot set, or indeed the von Koch snowflake (image via Wikipedia).

If you want a rectifiable example, if I recall correctly the definition of chord arc requires that the cord between two points has length comparable to their distance. So surely you can just take the graph of a function that oscillates near 0 in such a way that the individual pieces up to a given t will be summable (hence the curve is rectifiable) but the sum is not $O(t)$. For example, $$\gamma(t)=t+i|t|^{3/2}\sin(1/t)$$ ought to do it if I am not mistaken. Here it is shown (via graphsketch.com):

$\gamma(t)$" />

(EDIT 1. Images have been added below.)

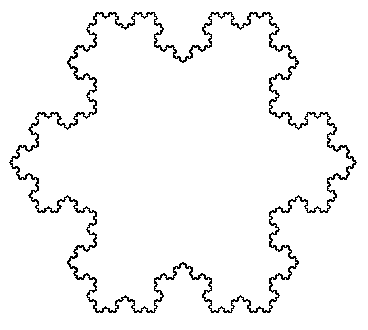
As far as I understand, any non-rectifiable curve is not chord arc. So any such curve will do, e.g. the Julia set of $z^2+c$ for $c\neq 0$ in the main cardioid of the Mandelbrot set, or indeed the von Koch snowflake (image via Wikipedia).

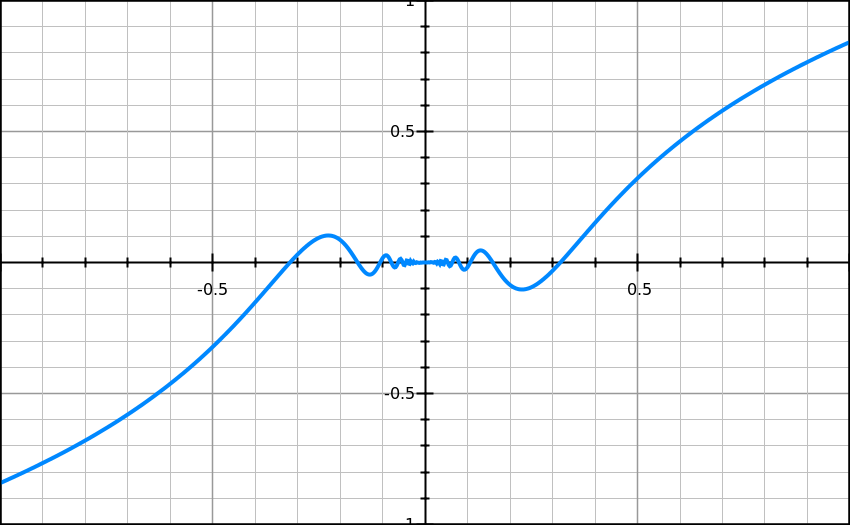
If you want a rectifiable example, if I recall correctly the definition of chord arc requires that the cord between two points has length comparable to their distance. So surely you can just take the graph of a function that oscillates near 0 in such a way that the individual pieces up to a given t will be summable (hence the curve is rectifiable) but the sum is not $O(t)$. For example, $$\gamma(t)=t+i|t|^{3/2}\sin(1/t)$$ ought to do it if I am not mistaken. Here it is shown (via graphsketch.com):

$\gamma(t)$" />

EDIT 2. You asked also about non-Smirnov domains. Assuming again that you want a boundary of finite length, this is much harder. There is a construction by Duren, Shapiro and Shields (Duke Math J, 1966), which is also explained by Pommerenke in his book "Boundary behaviour of conformal maps", Section 7.3. This construction is analytic in nature.

There was a previous example, by Keldysh and Lavrentiev (Ann. ENS, 1937). According to the Duren et al. paper, this example is based on a "complicated geometric construction". Øyma (PAMS, 1995) adapted this construction to show that, basically, any domain can be approximated by a non-Smirnov domain. He also cites a book by Privalev (Randeigenschaften analytischer Funktionen, 1956) for a detailed description of the construction of Keldysh and Lavrentiev. So you may find something there. According to Pommerenke's book, no geometric characterisation of Smirnov domains is known, and AFAIK that is still the case.

As far as I understand, any non-rectifiable curve is not chord arc. So any such curve will do, e.g. the Julia set of $z^2+c$ for $c\neq 0$ in the main cardioid of the Mandelbrot set.

If you want a rectifiable example, if I recall correctly the definition of chord arc requires that the cord between two points has length comparable to their distance. So surely you can just take the graph of a function that oscillates near 0 in such a way that the individual pieces up to a given t will be summable (hence the curve is rectifiable) but the sum is not $O(t)$. For example, $$t+it^{3/2}\sin(1/t)$$ ought to do it if I am not mistaken.

(EDIT. Images have been added below.)

As far as I understand, any non-rectifiable curve is not chord arc. So any such curve will do, e.g. the Julia set of $z^2+c$ for $c\neq 0$ in the main cardioid of the Mandelbrot set, or indeed the von Koch snowflake (image via Wikipedia).

If you want a rectifiable example, if I recall correctly the definition of chord arc requires that the cord between two points has length comparable to their distance. So surely you can just take the graph of a function that oscillates near 0 in such a way that the individual pieces up to a given t will be summable (hence the curve is rectifiable) but the sum is not $O(t)$. For example, $$\gamma(t)=t+i|t|^{3/2}\sin(1/t)$$ ought to do it if I am not mistaken. Here it is shown (via graphsketch.com):

$\gamma(t)$" />