I don't know any reference for this, and I don't know if this should be a "classical result", but let me give a lower bound, which might even be tight.
Let's denote the base of the cap by $BS$. It is a sphere of dimension $n-2$ with radius $$r=\sqrt{1-(1-\varepsilon)^2}=\sqrt{2\varepsilon-\varepsilon^2}.$$
Let $\Delta_{n-1}^R$ denote a regular simplex of dimension $n-1$ inscribed in a sphere of dimension $n-2$ with radius $R$. If I am not mistaken, the volume can be calculated as follows:
$$\text{vol}_{n-1}(\Delta_{n-1}^1)=\frac{n^\frac{n}{2}}{(n-1)^{\frac{n-1}{2}}(n-1)!}$$ and $$\text{vol}_{n-1}(\Delta_{n-1}^R)=R^{n-1}\text{vol}_{n-1}(\Delta_{n-1}^1).$$
Now if we define an $n$-simplex $P_n(\varepsilon)$ as the convex hull of the apex of the cap together with the vertices of a regular $(n-1)$-simplex $\Delta_{n-1}^r$ inside the sphere $\partial BS$, we can calculate its $n$-dimensional volume as follows: $$\begin{align}\text{vol}_n(P_n(\varepsilon))&=\frac{\varepsilon}{n}\text{vol}_{n-1}(\Delta_{n-1}^r)\\ &=\frac{\varepsilon}{n} r^{n-1}\text{vol}_{n-1}(\Delta_{n-1}^1)\\ &=\frac{\varepsilon}{n} (\sqrt{2\varepsilon-\varepsilon^2})^{n-1}\text{vol}_{n-1}(\Delta_{n-1}^1)\\ &=\frac{\varepsilon}{n} (\sqrt{2\varepsilon-\varepsilon^2})^{n-1}\frac{n^\frac{n}{2}}{(n-1)^{\frac{n-1}{2}}(n-1)!} \end{align} $$
Here is an illustration for $n=3$:
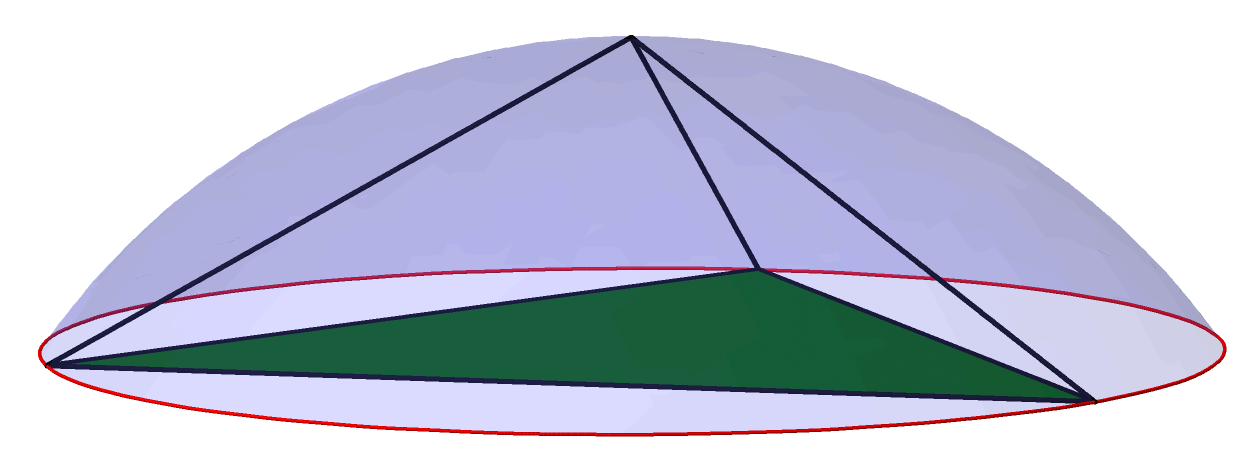This agrees with the order $\varepsilon^\frac{n+1}{2}$ for $\varepsilon\rightarrow 0$ that you expected and you can easily get complete asymptotics to all orders. Clearly this is a lower bound:
$$\Gamma\geq\text{vol}_n(P_n(\varepsilon)).$$
I find it plausible that this bound is tight. For this you would need to show two things are true for small $\varepsilon$:
- a largest simplex in the cap has all but one vertex in the base of the cap (see comment by Joseph O'Rourke.)
- for a largest simplex those vertices in the base of the cap form a regular simplex.
Here is an illustration for $n=3$: