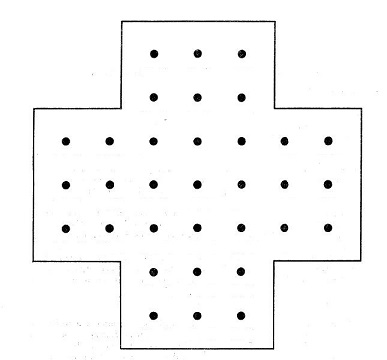
Peg solitaire which I played on chess board in childhood. Let me explain this solitary game; consider the following table consisted of 33 holes.
There is a peg in all except one of the holes.(Assume that one is in the center). You can move a peg horizontally and vertically. The permissible move consists of jumping one peg over another into an empty hole and simultaneously removing the peg has been jumped over. One can continue this process to reach a situation than cannot have an acceptable move. And the player is winner if the last situation has only one peg.
Thus we can ask some question:
Does this play has a winning strategy?
What are the possible last situations?
The interesting point is the analysis of this game relates to the finite field with 4 elements, GF(4). The answer to question 1 is yes. For question 2 please consult the papaerpaper "A solitaire game and its relation to a finite field" by N. G. de Bruijin.
It seems the result of the paper can be extended. I think it is useful in a course in algebra, I exposed the paper when I was a TA in algebra 2.