There is a related iterated function system with two functions,
$f_0(x) = 1+zx$
$f_1(x) = -1+zx$$f_1(x) = -1+zx.$
$X_z$ is the unique nonempty compact fixed set of this iterated function system. It is sometimes called a generalized dragon set with parameter $z$, and particular values of $z$ can produce some well-known fractals called dragons.
A relevant result on iterated function systems is that the fixed set $X$ is connected iff it is arcwise connected iff the family of subsets $\{f_i(X)\}$ is connected, which in this case means $f_0(X_z) \cap f_1(X_z)$ is nonempty. (This paper refers to Kigami, Analysis on Fractals chapter 1 for the result.) So, the set is connected iff we can write
$$\begin{eqnarray} 1 + \sum_{i=1}^{\infty} a_i z^i &=& -1 + \sum_{i=1}^\infty a_i' z^i \newline 1 + \sum_{i=1}^{\infty} \frac{a_i-a_i'}{2} z^i &=& 0 \newline 1 + \sum_{i=1}^{\infty} b_i z^i &=& 0 \end{eqnarray}$$
where $b_i = (a_i-a_i')/2 \in \{0,1,-1\}$.
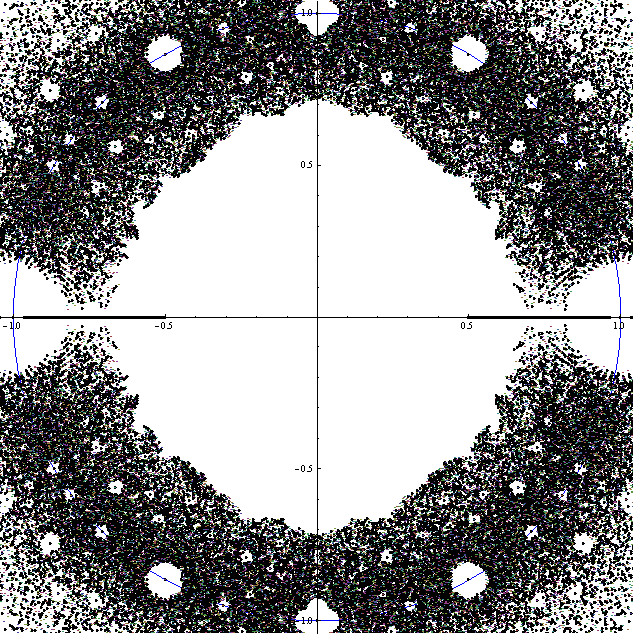
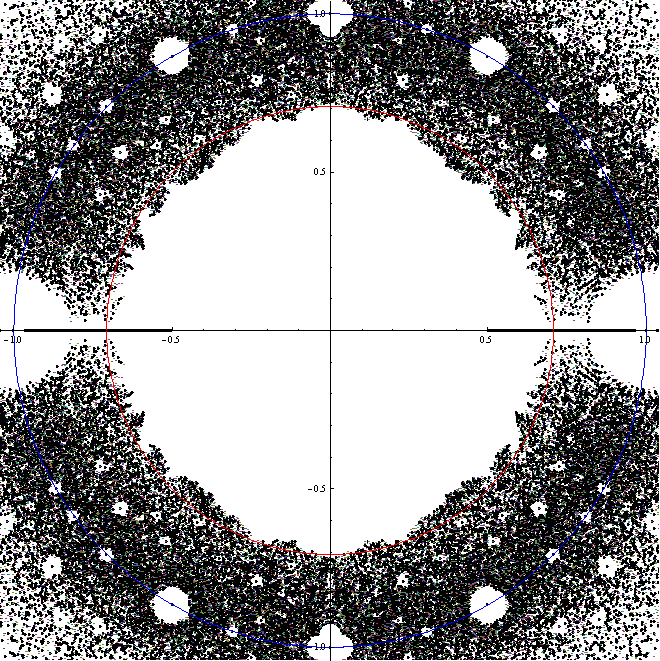
In particular, $X_z$ is connected when $z$ is real with $1/2 \le |z| \lt 1$ and when $z$ is a root of a polynomial with coefficients in $\{-1,0,1\}$. The intersection of the closure of those roots with the interior of the disk is the entire set where $X_z$ is connected$M$.
This image shows the nonzero roots of polynomials of degrees up to $9$ with coefficients in $\{-1,0,1\}$ with the unit circlecircles of radii $\frac{1}{\sqrt{2}}$ and $1$.
The closures of roots of polynomials with restricted coefficients have been studied, and they are quite interesting. In some areas, there seems to be a Julia-Mandelbrot correspondence, where the set of roots of small degree near a point resembles the fixed set of the iterated function at that point. Edit: I misread thisThis preprint of Thierry Bousch earlier. It appears relevant, provingproves some connectivity properties of the closure, and that the annulus $\frac{1}{\sqrt{2}} \lt |z| \lt 1$ is in $M$. So, some of the apparent holes in the picture above close up as the degree increases, including those between the two circles such as near some roots of unity. The paper of Calegari et al mentioned by Nikita Sidorov supercedes it regarding the structure of the complementproves that there are many actual holes in $M$, among other results.