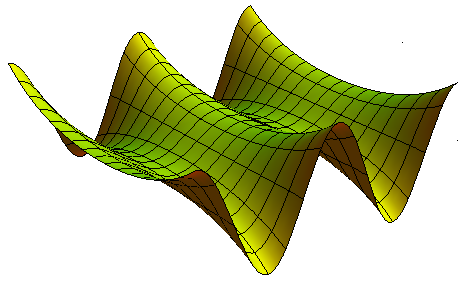
We get negative Gauss curvature K surfaces of quasi polar symmetry surface form generated from:
$$ Re (x+ i y)^n = a^n $$ (n integer) with n humps above plane $ z =0$.
($ n =2,3,4 $ hyperbolic paraboloids, monkey saddles, four humped frill/ pleated shells respectively).
"Quasi" due to $n>2$ introducing circumferential pleats or frills.
What polynomial or differential relation function yields constant negative surfaces of $K = -1/a^2 $?
How to set up its differential equation?
Instead of $ z^n $ if a simpler $ \cos z $ is taken, no clue or handle how to modify the function in order to get a constant negative K.$ K. ( \cos x \cosh y = $ const)