You can express the vector $\mathcal{N}_{i,p} = (N_{i-p,p}(x), \ldots, N_{i,p}(x))$ as a product of certain matrices $R_1\cdots R_p$, where
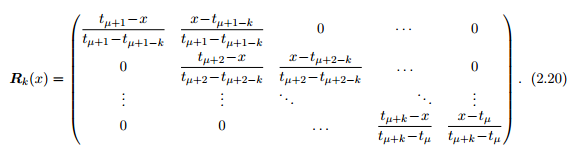
(here $\mu =i$ and $t_j = u_j$)
These matrices have a very neat property: $R_k(x)' R_{k+1}(x) = R_k(x) R_{k+1}(x)'$ which allows you to simplify the result of the Leibniz rule $$ \frac{d}{dx} \mathcal{N}_{i,p} = \sum_{k=1}^p R_1(x)\cdots R_{k-1}(x) R_k(x)' R_{k+1}(x)\cdots R_p(x) $$ which gives you $$ \frac{d}{dx} \mathcal{N}_{i,p} (x) = p \mathcal{N}_{i,p-1} (x) R_d'(x). $$ Then you just calculate the derivative of the matrix $R_d$ and compare left hand side with the right hand side.
