In the book The unstable Adams spectral sequence for free iterated loop spaces, R.J. Wellington, Mem. Amer. Math. Soc. 258, 1982, p. 32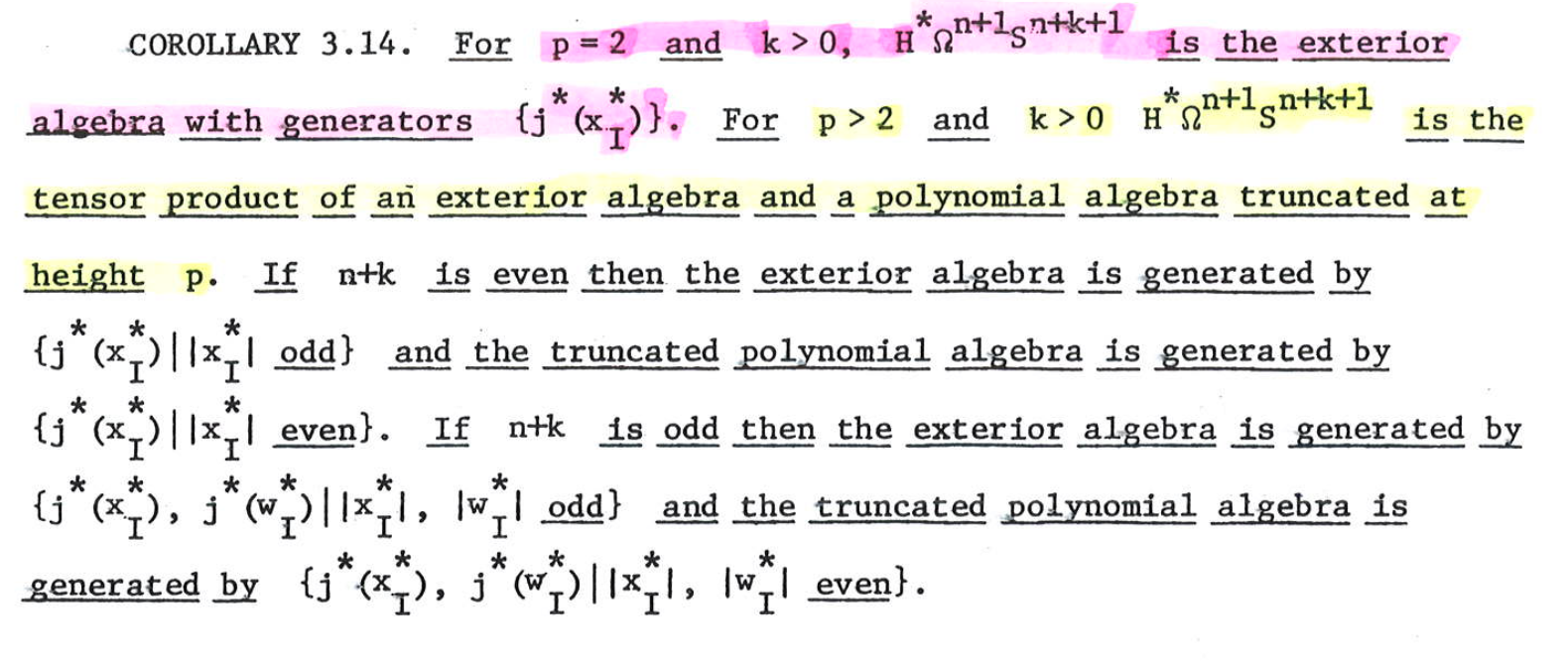
Question: When $p=2$, $k\geq 2$ and$k\geq 1$, $n=\infty$$n=0$ to $\infty$, what is the dimensionkind of each generator $j^*(x_I^*)$$I$ can we choose? I do not quite understandHow is the argument.exterior algebra $H^*(\Omega^{n+1}\Omega^{n+k+1};\mathbb{Z}_2)$ related to $n$?
The related context is in the following.


