This is a crosspost of this MSE questionthis MSE question according to the recommendation in the comments. I know this question is elementary, but I'm hoping the author of these notes could provide a more detailed explanation.
Here is an excerpt from some notes I stumbled upon online:
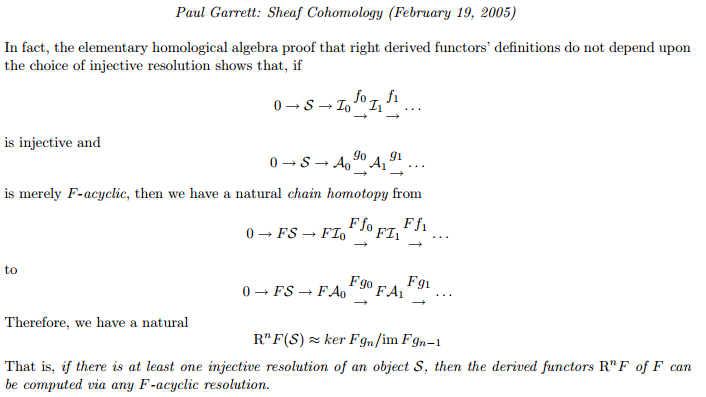
From what I understand, the "elementary proof" is just the fundamental lemma of homological algebra which says the homotopy type of chain maps out of projective resolutions is determined by maps between the objects being resolved.
It seems that the author says that the image of every $F$-acyclic resolution is homotopic to some injective/projective resolution, but I don't think this follows from the fundamental lemma.
Why is this true? How does it prove one may compute derived functors using $F$-acyclics? Does this approach circumvent dimension shifting?
