Formal vs self-organised knowledge systems: a network approach
A.P. Masucci, Physica A 390 (2011) 4652-4659 (arXiv:1105.1058)
In this work we consider the topological analysis of symbolic formal systems in the framework of network theory. In particular we analyse the network extracted by Principia Mathematica of B. Russell and A.N. Whitehead, where the vertices are the statements and two statements are connected with a directed link if one statement is used to demonstrate the other one. We compare the obtained network with other directed acyclic graphs, such as a scientific citation network and a stochastic model. We also introduce a novel topological ordering for directed acyclic graphs and we discuss its properties in respect to the classical one. The main result is the observation that formal systems of knowledge topologically behave similarly to self-organised systems.
This paper looks at how logical statements such as theorems are organized in specific expository works (like the Principia). As others have noted in the comments, outside such organized works, the dependencies can be ambiguous as theorems can have alternate proofs. Moreover, the order of deriving theorems is not canonical so that if all proof paths were included, cycles will have formed. The Principia is as good as any work to start with, but I would really love to see a similar analysis on Bourbaki and on Euclid's Elements.
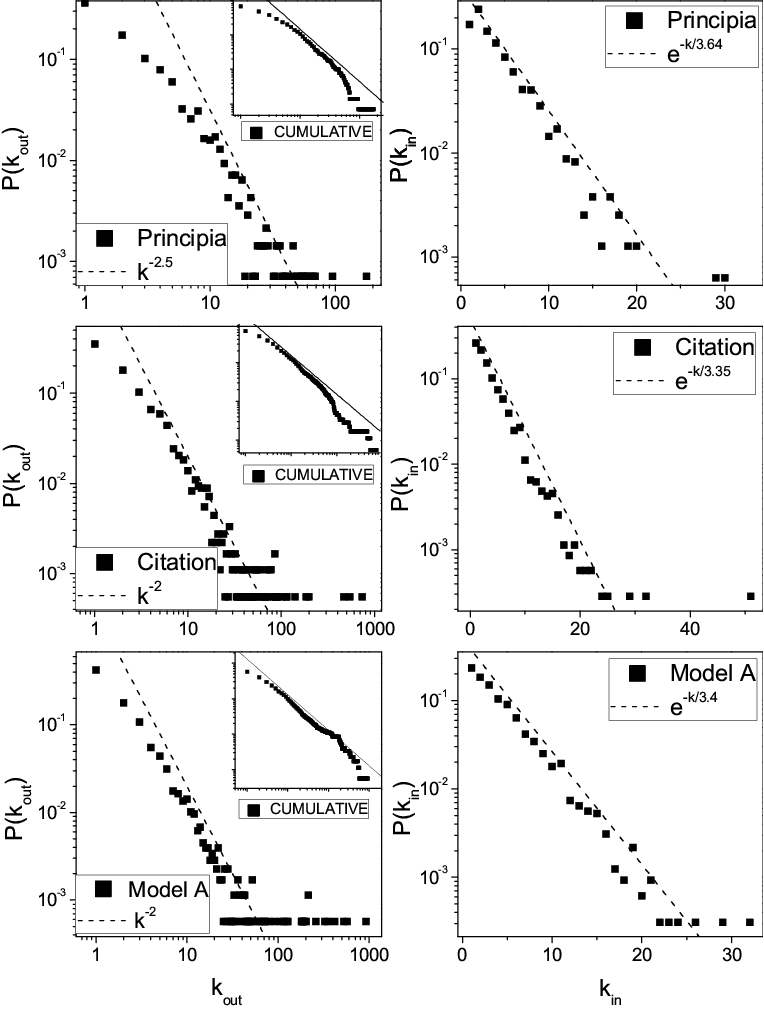 Figure from the paper: distribution of in and out degrees in the Principia DAG, in the DAG of citations, and in a stochastic generative model.
Figure from the paper: distribution of in and out degrees in the Principia DAG, in the DAG of citations, and in a stochastic generative model.
