Let
$$I_{n,p,a}:=\int_0^{\infty-} \frac{g_{n,a}(t)}{t^{p+1}} \, dt,$$
where
$$(*)\qquad\qquad\qquad n\in\mathbb N,\quad -\infty<a<\infty,\quad p_{n,a} < p < n,\qquad\qquad\qquad\qquad\phantom{x} $$
$p_{n,a}$ equals $0$ or $-1$ depending on whether $a$ belongs to the set $\{2j-n\colon\, j=0,\dots,n\}$ or not,
and
$$g_{n,a}(t):=e^{i a t} \left(e^{i t}-e^{-i t}\right)^n.$$
The integral $I_{n,p,a}$ arises in certain explicit expressions of certain linear functionals of a measure in terms of the Fourier transform of the measure. It is not hard to see that for non-integer $p\in(p_{n,a},n)$
$$I_{n,p,a}=i^p\, \Gamma (-p)\, \sum _{j=0}^n (-1)^j \binom{n}{j}\, \overline{(2j-n-a)^p},$$
where $\overline{z}$ denotes the complex conjugate of $z$, and $I_{n,p,a}$ is continuous in $p\in(p_{n,a},n)$, so that the values of $I_{n,p,a}$ in the case when $p$ is an integer in the interval $(p_{n,a},n)$ can be obtained by the l'Hospital rule.
Here we follow the usual convention that the (principal value of the) argument $\arg z$ of a nonzero complex number $z$ belongs to the interval $(-\pi,\pi]$, and then $z^p$ is understood as $|z|^p e^{ip\arg z}$.
The question is this: Do there exist $n$, $p$, and $a$ such that conditions $(*)$ hold and $I_{n,p,a}=0$? It appears that the answer is negative.
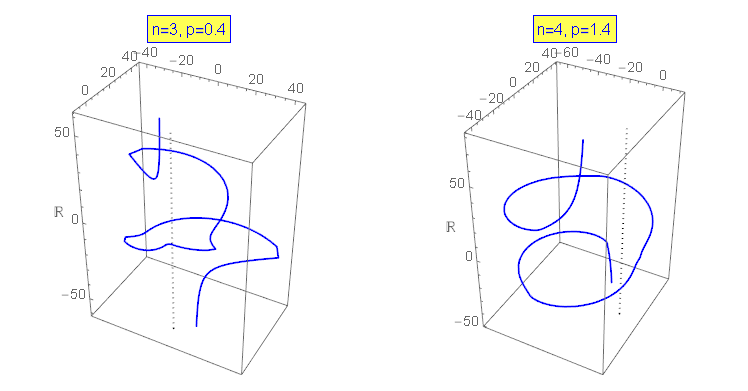
Below are pictures of the sets $\{(I_{3,\,-0.4,\,a},\,10a)\colon -2\pi<a<3\pi\}$ and$\big\{\big((1 + a^2)^{(n - p)/2}I_{n,p,\,a},\,6a\big)\colon -10<a<10\big\}$ for $(n,p)=(3,0.4)$ and $\{(I_{4,\,1.4,\,a},\,a)\colon -2\pi<a<3\pi\}$$\big\{\big((1 + a^2)^{(n - p)/2}I_{n,p,a},\,10a\big)\colon -6<a<10\big\}$ for $(n,p)=(4,1.4)$ in $\mathbb{C}\times\mathbb R$. In particular In each picture, they suggest thatshown also is the $I_{n,p,a}\to0$ as(dotted) vertical line through the point $a\to\pm\infty$$0\in\mathbb C$, as it of course must berespectively $\big\{(0,\,6a\big)\colon -10<a<10\big\}$ for $(n,p)=(3,0.4)$ and $\big\{(0,\,10a\big)\colon -6<a<10\big\}$ for $(n,p)=(4,1.4)$. The factor $\big((1 + a^2)^{(n - p)/2}$ at $I_{n,p,a}$ is in accordance with the expression for $I_{n,p,a}$ given in the answer in the case when $|a|>n$.