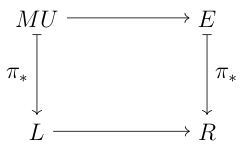
Recall that a complex-oriented spectrum is a ring spectrum E with a map $MU \to E$.
Analogously, a ring with a (1-d commutative) formal group law is (represented by) a ring $R$ with a map $L \to R$ (where $L$ is the Lazard ring).
In the cases where $L \to R$ is Landweber exact, this can be made explicit:
However, the explicit lifting condition can't just be Landweber exactness. For example: the additive formal group law, though not Landweber-exact, corresponds to $H\mathbb{Z}$; the $n$th Honda formal group law, though not Landweber-exact, corresponds to $K(n)$.
My question is not "are there rings with (1-d commutative) formal group laws for which there is no corresponding map between ring spectra," but instead along the lines of "how do I build an explicit example."
Edit: A stupid explicit example of a formal group law that doesn't lift to a complex-orientable spectrum is any formal group law over $\mathbb{F}_p$ that is not isomorphic to the additive formal group law over $\mathbb{F}_p$. The only complex orientable spectra associated to formal group laws over $\mathbb{F}_p$ is $H\mathbb{F}_p$ (everything is concentrated in one degree). However, we can of course have a formal group law over $\mathbb{F}_p[[u_n, u_n^{-1}]]$ which lifts to a periodic ring spectrum.
What are explicit obstructions to realizability of formal group laws as complex-oriented ring spectra?
A vague guess is that the difference might come from the algebra of the ring $MU^*$ behaving differently from the 'homotopical' algebra of the ring spectrum $MU$, but I'm not sure how to proceed from this.
