I have a random closed curve of the form $(\theta,r_\theta)$, where $\theta\in [0,2\pi]$, is the counter clockwise angle from the x-axis and $r(\theta)$$r_\theta$ is the radial distance from the origin (centroid).
For example:
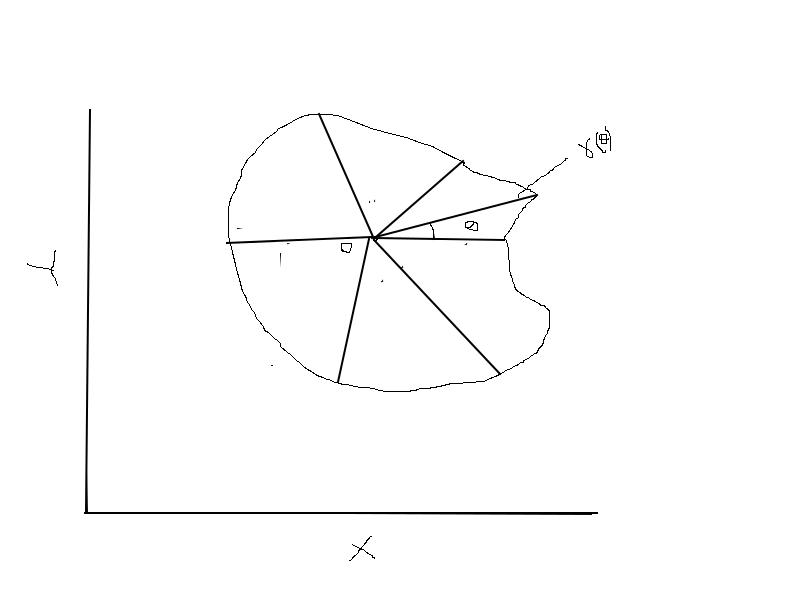
Is it possible to define a stochastic process $r=\{r_\theta: \theta \in [0,2\pi]\}$ as a Brownian bridge?
