Two recommendations:
Senechal, Marjorie. Quasicrystals and geometry. Cambriged Univ Press, 1996. Review by Charles Radin in the AMS Notices: PDF download.
Baake, Michael. "A guide to mathematical quasicrystals." Quasicrystals. Springer Berlin Heidelberg, 2002. 17-48. (arXiv prepub link.)
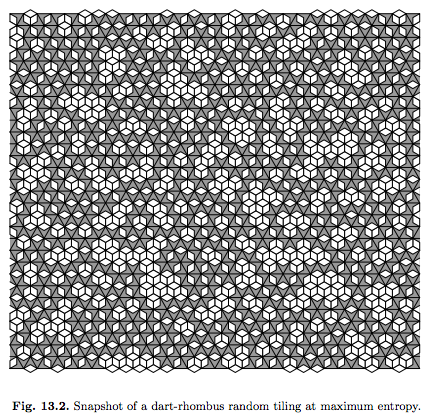
Maximum entropy equals $\frac{1}{3} \log 2$.

