If we have 3 circles on the plane with tangent lines, we can notice they have colinear intersection!
Made in inkscape http://web.archive.org/web/20131103103255/http://img256.imageshack.us/img256/7512/picture1dt.png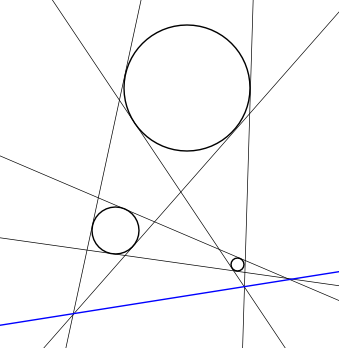
To prove it, we can visualize the same configuration in 3D, the balls lay on a surface and rather than tangent lines we take cones: The colinearity comes from the fact that if we lay a plane ontop of this configuration it will intersect the table in a line!
This is from 'curious and interesting geometry' and the proof is attributed to John Edson Sweet. I really like this proof because it gives a vivid example of the general idea that sometimes, to solve a problem in the most simple way you need to view it as a part of some bigger whole.
