This is not my answer originally (see comments above) so I'm making it community wiki. Just among pulled triangulations of the 3-cube (that is, triangulations formed by choosing a vertex, triangulating the squares of the cube that do not touch this vertex, and connecting each of these triangles to the chosen vertex) there are eight choices of vertex to pull, and eight choices of how to triangulate. As TheMaskedAvenger has described, these may also be described by partitioning the cube into three square pyramids and then triangulating each pyramid in one of two ways, as shown below.
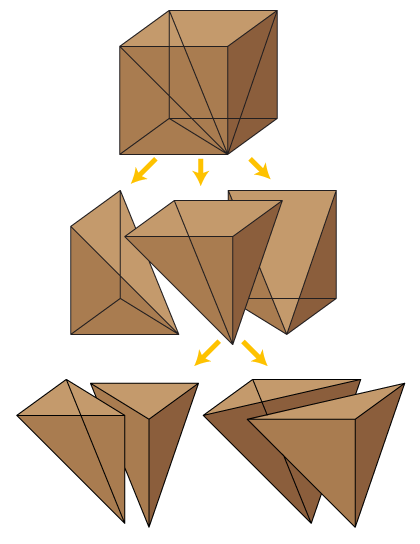
There are 60 of these pulled triangulations, not 64, because for each chosen vertex one of the triangulations is the same as one where the opposite vertex is chosen.
There are also 12 more triangulations of the 3-cube that are a little trickier to describe. Choose one of the four triangulations that are pulled from two opposite vertices. Then, choose two opposite square faces of the cube, merge the two tetrahedra that touch that face, and split the resulting two pyramids in the other way. So altogether the cube has at least 72 triangulations.
In 4d, you can then do a pulling triangulation again, choosing one of these 6072 triangulations in each of the four 3-cube facets that are opposite the chosen vertex. You can't choose them independently (if we're forming triangulations rather than partitions) because the diagonals have to match on the squares connecting these 3-cubes. Even so the number of possible triangulations seems to grows very quickly with the dimension...
