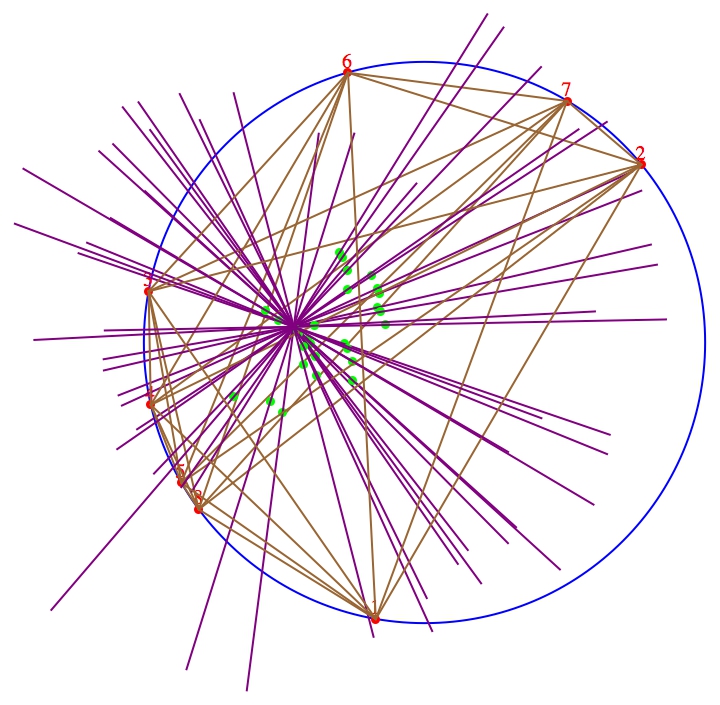
As this construction was new to me, I wanted to see it. Here are two random examples. The green points are centroids of the triples of points.
http://cs.smith.edu/~orourke/MathOverflow/FivePointsr34.jpg
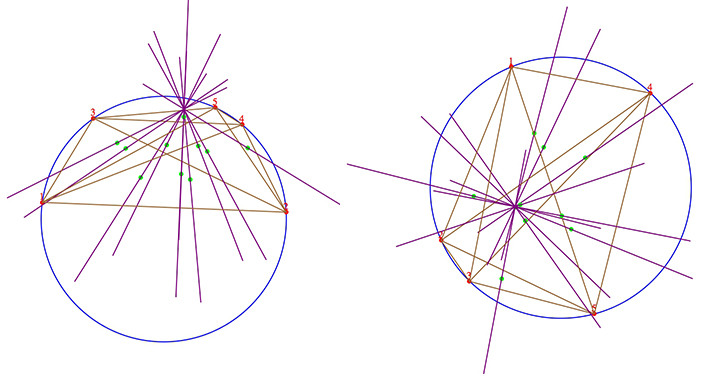
Concerning the question, "Can the circle be generalized to any conic?": certainly not straightforwardly.
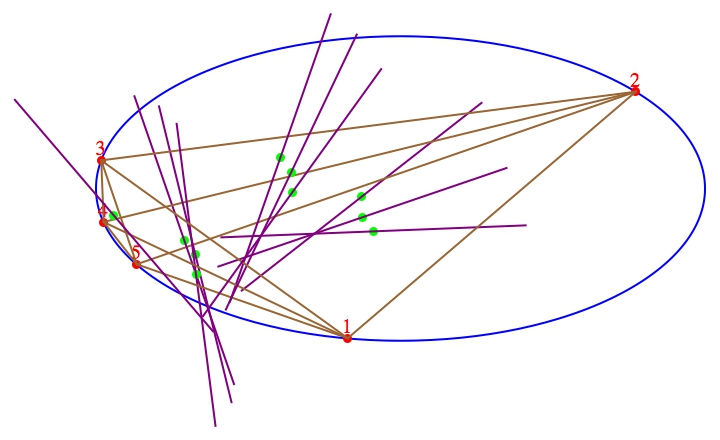
Update. Here is an illustration of Aaron Meyerowitz's beautiful theorem, for $N=8$, $m=2$, showing coincidence of the $\binom{8}{2}=28$ lines through the $n=6$ hexagon centroids $q$ (green) and (in this case) perpendicular to the line through the two complementary points: