I think the proof of @domotrop is not complete.
I will first present my own proof. After several revisions, it is now complete. Then I will express my concern for the proof of @domotrop, and propose a fix.
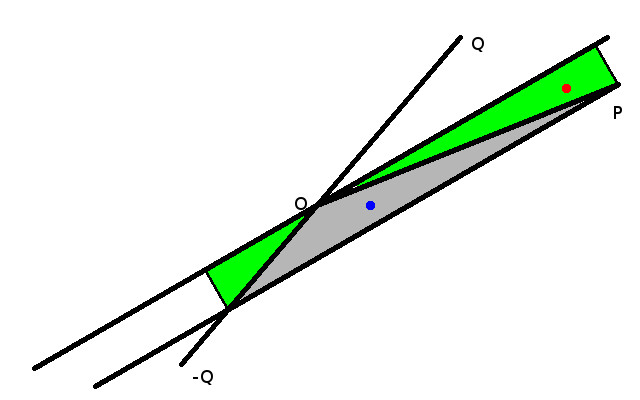
In the following picture, $O$ is the origin. Let $P$ and $Q$ be the closest points with angle $\theta_k$ and $\theta_{k+1}$. The point $-Q$ is the opposite of $Q$. Without loss of generality, we assume that $P$ is closer to $\ell$ than $Q$.
Up to a change of sign, any closer lattice point with larger angle from $\ell$ will be in the grey area, for example the blue point. Then with the help of a parallelogram, we can find the red lattice point in either of the green areas with smaller angle from $\ell$. This red point is definitely inside the circle, so it contradicts our assumption.
I think the proof of @domotrop is not complete. My concern for @domotrop's proof was the following: For example, in the picture of @Rob, $P$ minimize the angle with $\ell$ from below, but the point $(-1,-1)$ is closer with a larger angle from $\ell$. Indeed it corresponds to $(1,1)$, a closer point on the other side in the upper half space, but I'm not sure that $(1,1)$ minimize the angle from above. Therefore, if $\arg(n+im)$ takes value in $(-\pi,\pi]$, the points like $(-1,-1)$, which are in the lower half-space, is something that we should be careful with. That is, either there is no such point, or the opposite of the closest is indeed the next angle.
The fix consists of considering the opposite of the $\theta_{k+1}$ as in my proof. Then, in the above picture, since the green parts are empty, so is the grey part, and Q.E.D. @domotrop explained that we only needs to consider the two quadrants containing $\ell$. Then his proof is complete after repeating the same argument above $\ell$ in the first quadrant, and replacing $y$ with $x$ when constructing the parallelogram.
