For each $\alpha \in S^1$, the map $\varphi_{\alpha} \colon \mathbb Z \to S^1$, given by $n \mapsto \alpha^n$, induces a topology $\tau_{\alpha}$ on $\mathbb Z$.
A basis of neighborhoods of $0$ for $\tau_{\alpha}$ is given by the sets $$U_{n,\alpha} := \left\{k \in \mathbb Z \mid |\alpha^k-1| < \frac1n \right\}, \quad n \in \mathbb N.$$$$U_{n,\alpha} := \left\{k \in \mathbb Z \mid |\alpha^k-1| < \frac1n \right\}, \quad n \in \mathbb N.$$
I denote by $\tau_{\alpha} \wedge \tau_{\beta}$ the largest group topology that is contained in $\tau_{\alpha} \cap \tau_{\beta}$ - which is in general different from $\tau_{\alpha} \cap \tau_{\beta}$.
Claim 1: If $\alpha,\beta \in S^1$ are irrational and such that $\alpha/\beta \in S^1$ is also an irrational angle, then $\tau_{\alpha} \cap \tau_{\beta}$$\tau_{\alpha} \wedge \tau_{\beta}$ is trivial.
Proof: Let $U \in \tau_{\alpha} \cap \tau_{\beta}$$U \in \tau_{\alpha} \wedge \tau_{\beta} \subset \tau_{\alpha} \cap \tau_{\beta}$ and $0 \in U$. There exists some $V \in \tau_{\alpha} \cap \tau_{\beta}$$V \in \tau_{\alpha} \wedge \tau_{\beta}$ such that $V-V \subset U$ and $0 \in V$. Then, there exists $n$, such that $U_{n,\alpha} - U_{n,\beta} \subset V - V \subset U$. However, $\mathbb Z=U_{n,\alpha} - U_{n,\beta}$. Indeed, for any $k \in \mathbb Z$, there exists some $m$, such that $|\alpha^m-1|<\frac1n$ and $|\beta^{k+m}-1|=|\beta^{m} - \beta^{-k}|<\frac1n$, because $(\alpha,\beta)$ generates a dense subgroup of $S^1 \times S^1$ and thus can approximate the point $(1,\beta^{-k})$ arbitrarily well. q.e.d.
Claim 2: $\tau_{\alpha} \vee \tau_{\beta}$ is the topology induced from the map $\mathbb Z \to S^1 \times S^1$, $n \mapsto (\alpha^n,\beta^n)$.
In particular, applying various automorphisms of $S^1 \times S^1$, we see that $$\tau_{\alpha} \vee \tau_{\beta} = \tau_{\alpha} \vee \tau_{\alpha\beta} = \tau_{\beta} \vee \tau_{\alpha\beta}.$$
Let $\alpha,\beta \in S^1$ be irrational angles, such that $\alpha/\beta$ is also irrational and consider the topologies $\tau_{\alpha},\tau_{\beta},\tau_{\alpha \beta}$. Any pairwise intersectionmeet (in the lattice of group topologies) is the trivial topology (by Claim 1) and any pairwise join yields the same topology (by Claim 2). Hence,
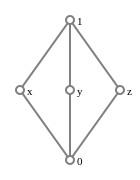
embeds as a sub-lattice in the lattice of group topologies. Hence, the lattice of group topologies cannot be distributive.
