Your definition of 2-reptile does not explicitly require that the two pieces are are congruent to each other, only that each is similar to the union. However you probably intended that and you got a good answer (which I was glad to learn about).
If we merely require that the two parts are similar to the whole then we have any right triangle divided by the altitude to the hypotenuse, however no rectangles.
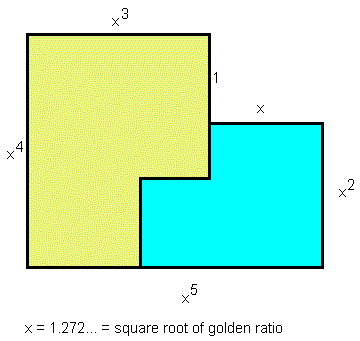
I convinced myself that there were no L-shaped examples. Then I used Google and found onefound one with scaling by $\sqrt{\varphi}$ for $\varphi=\frac{1+\sqrt5}{2}$:
![LL][2]