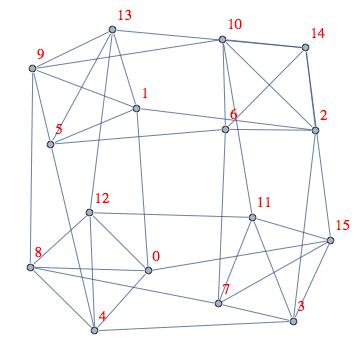
Not an answer; just a "public service," because I wanted to see Adam's example explicitly.
Here is $C_{16}(1,4,8)$ [if I computed this correctly]. So $1$ is connected to $(13,9,5,2)$ because $$16-|1{-}13|=4$$ $$16-|1{-}9|=8$$ $$|1{-}9|=8$$ $$|1{-}5|=4$$ $$|1{-}2|=1$$ Etc.: