In his 1939 book Weyl indeed did not use the notation SO but $O^+$, and called it the proper orthogonal group, but he did use the notation SU, or more precisely $^s$U with a tiny superscript s.
http://ilorentz.org/beenakker/MO/sU.png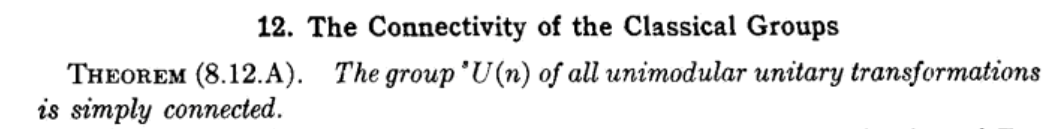
The name "special unitary group" does not appear, it is always "unimodular".
The footnote on the name "symplectic group", from the same book, is amusing:
http://ilorentz.org/beenakker/MO/symplectic.png
