This question loosely builds on this onethis one, however is a bit simpler and I found the results to be more robust.
It seems that all zeros in the critical strip $0 \lt \Re(s) < 1$ of:
$$Li_s(z)\, \pm \, Li_{1-s}(z)$$
reside on the critical line $\Re(s)=\frac12$ for all $z \le -1$.
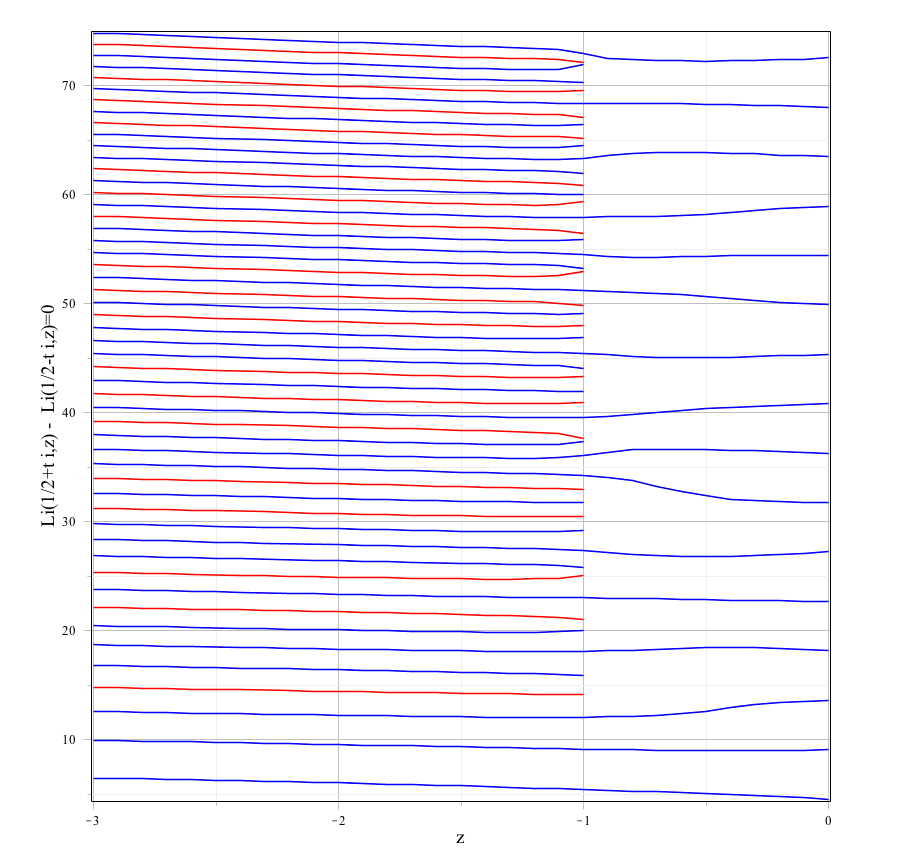
Below is a graph that shows where this function (using $\pm = -$) vanishes for $s=\frac12 \pm t\,i$ at different values of $z$. The lines marked in red all lead to a non-trivial zero of $\zeta(s)$, since $Li_s(-1)=\eta(s)$, with $\eta(s)$ being the Dirichlet Eta-function.
I have extended the graph towards $z \rightarrow 0^-$ to also show how some lines continue in that domain, however there clearly are zeros off the critical line when $z > -1$ (note: the lines coming from the left don't have a 'hard stop' at $z=-1$ and actually continue a short bit further to the right).
For the root finding process I used the following expression of the PolyLog function that I found to be evaluating much faster for higher values than the standard PolyLog function (in Maple):
$$Li_s(z) = \frac{\Gamma(1-s)}{(2\,\pi)^{1-s}} \left(i^{1-s}\,\zeta_H\left(1-s,\frac12+\frac{\ln(-z)}{2 \,\pi \, i}\right)+i^{s-1}\,\zeta_H\left(1-s,\frac12-\frac{\ln(-z)}{2 \,\pi \, i}\right)\right)$$
where $\zeta_H(s,q)$ is the Hurwitz zeta function.
Addition:
Numerical evidence suggests that the claim could be extended further into:
All complex zeros in the critical strip $0 \lt \Re(s) < 1$ of:
$$Li_s(z)\, \pm \, Li_{2a-s}(z)$$
reside on the critical line $\Re(s)=a$ for all $z \le -1$ and all $a \le \frac12$ (this might be related to this paper).
The patterns of lines of zeros at $s=a + t i$ are very similar to the graph above and values of $t$ only shift slightly at different $a$. However, the zeros at $z \rightarrow 0^-$ always remain $s=a \pm \frac{k \, \pi \, i}{\ln(2)},k \in \mathbb{N}$ and their imaginary part $t$ seems independent of the choice of $a$. This might be a clue for answering my second question (note that $2^s-2^{2a-s}$ has the same roots, however I did not find any connection).
Questions:
Is there a counterexample with a zero lying off the critical line, but in the critical strip for $z \le -1$?
When $z \rightarrow 0^-$ the function $Li_s(z)\, - \, Li_{1-s}(z)$ vanishes at $s=\frac12 \pm \frac{k \, \pi \, i}{\ln(2)}$ with $k \in \mathbb{N}$, however I failed to derive this apparently trivial result from the known formulae. Could this be proven?
Thanks.
