Let n$n$ be a natural number. Let dc(n)$dc(n)$ be the number of compositionscompositions of n$n$ where the summands are required to be in the set of divisors of n$n$. Standard lore in analytic combinatorics yields the following formula for dc(n)$dc(n)$:
dc(n) = nth Taylor coefficient of 1/(1- \sum_{m ∈ divisors of n} z^m)
$$dc(n) = n\text{th Taylor coefficient of }\frac{1}{1- \sum_{m\in\text{divisors of n}} z^m}.$$
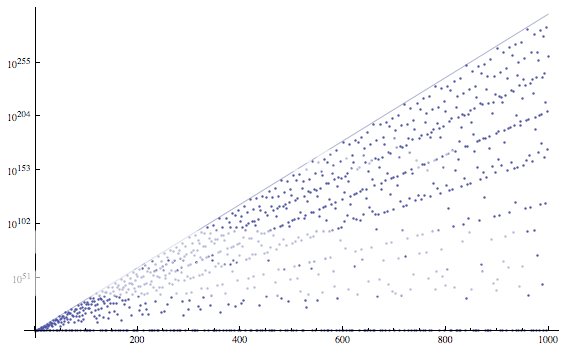
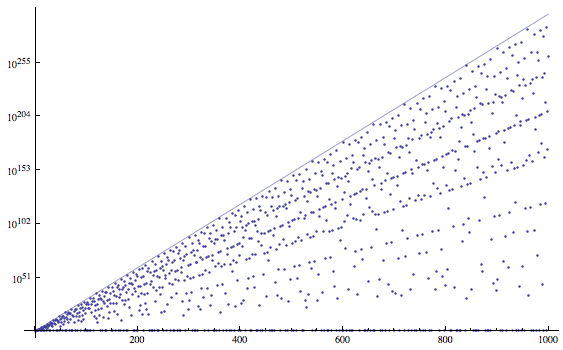
But what are the asymptotics of dc(n)$dc(n)$? Here's a plot that I made (the y-axis is dc(n)$dc(n)$ on a log scale and the x-axis is n$n$):
I would like to understand the "fanning", which presumably has something to do with whether numbers have lots of small divisors or not, and I would also like to understand why these fans seem to be so close to exponentials. The solid fit line at the top is 2^n$2^n$, which is the number of unrestricted compositions.
If that's too much to ask for, I guess I'd like to know how one might use known facts about the number of divisors, etc. to say something about this, as this rather artificial construction ought to be governed by some more fundamental number theoretic functions.