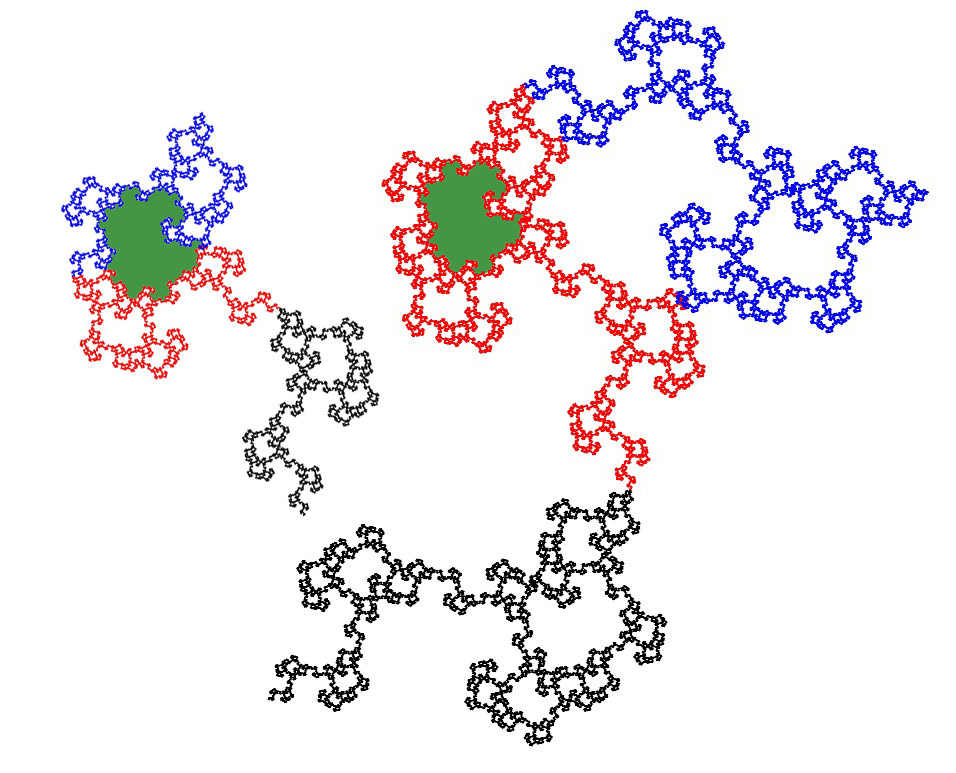
In your first example, I think the ratio is $$ \arctan\frac{\sqrt{127+96\sqrt{2}\;}}{7} \approx 66.643774 \text{ degrees} $$ There is no reason to think this is a rational number of degrees. And: the second hole is exactly half the size of the largest hole!
These problems are fun...
more information
Two of the methods to study self-similar sets are: the L-System (Lindenmayer System) and the IFS (iterated function system). To analyze this L-System, I first had to get the corresponding IFS. (Perhaps there are others who, to analyze an IFS, first convert it to an L-System...)
Here it is:
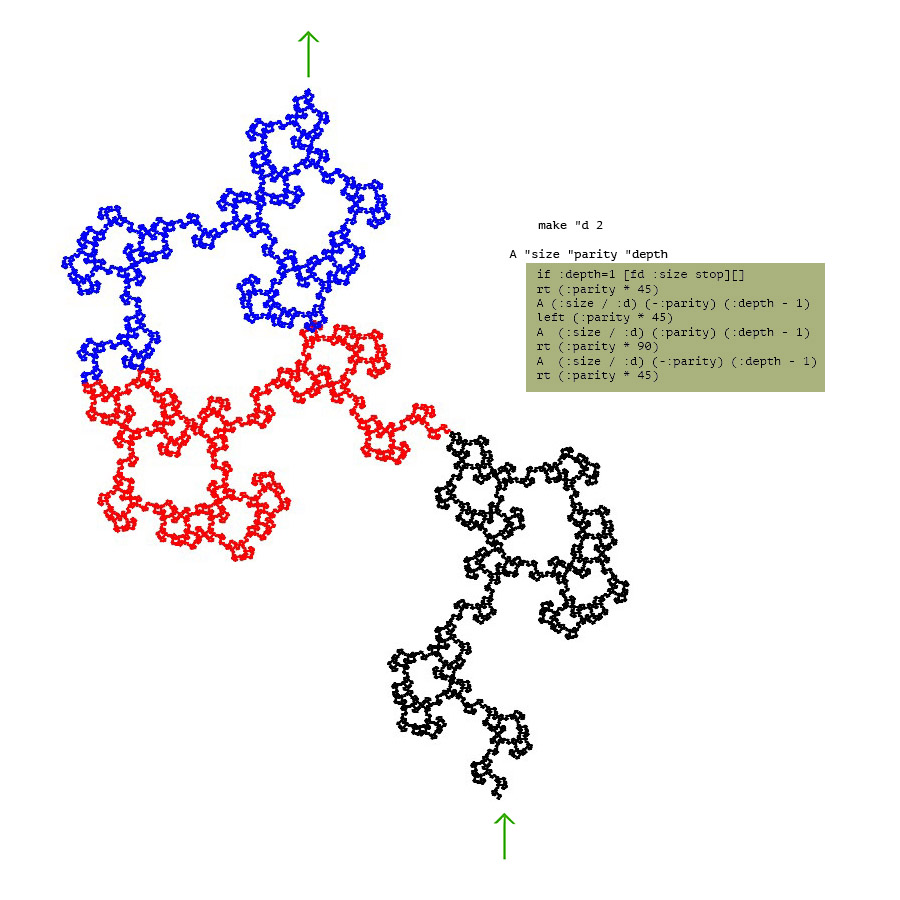
The factor $d=2$, comparing the size of the whole to the size of one of the three parts, was found by trial-and-error. If you make $d$ too large or too small, then higher iterates of the picture either grow without bound, or shrink to zero. But factor $2$ seems to keep them about the same size. The one in the picture shows what it looks like when depth is odd. The whole thing is made up of three parts, similar to the original (the first and third reflected). The orientation at the exit is the same as the orientation at the entry.
For even depth, the picture looks like this:
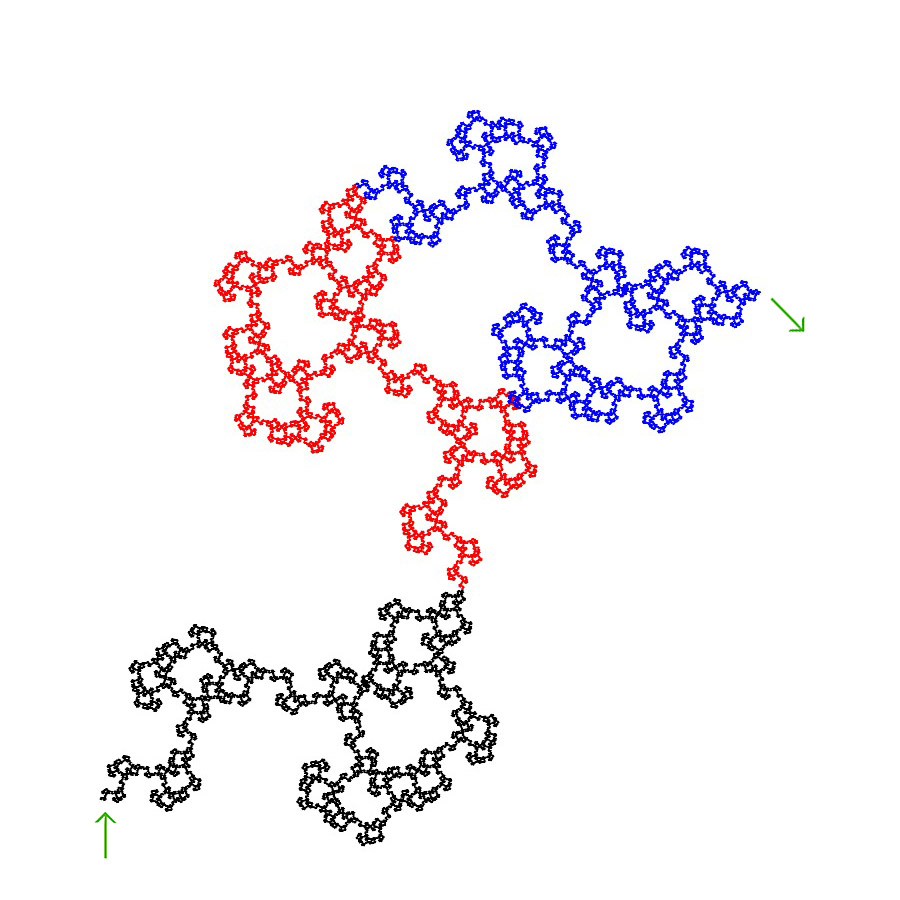
It looks exactly like an odd case, except rotated by a certain amount. (It turns out, rotated by that approximate 66 degrees described above.) And the exit orientation is rotated $-3\pi/4$ clockwise from the entry orientation.
Now, in the odd cases, the direction from entry point to exit point differs from the entry direction by some angle $\alpha$. In the even cases, the direction from entry pooint to exit point differs from a $-\pi/2$ direction by some angle $-\beta$.
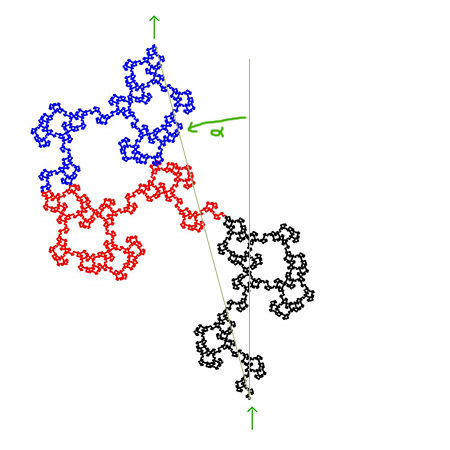
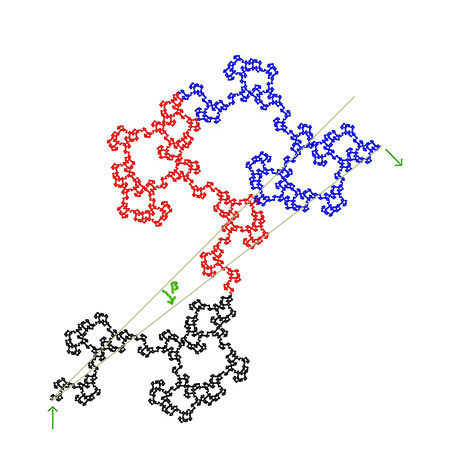
So let's analyze the odd picture into its three parts (which are even pictures, some reflected). (This picture is rotated $-\pi/2$ since I want to use complex numbers to do my trigonometry: the entry direction is complex number $1$, represented as a vector pointing to the right.)
To get from the entry point to the exit point of the whole thing (let's normalize so that this distance is $1$ unit), we go $1/2$ unit in direction $\beta$, then $1/2$ unit in direction $\pi/2-\beta$, then $1/2$ unit in direction $-\pi/4 + \beta$. So (starting at $0$) the exit point is $$ w:=\frac{e^{i\beta}+e^{i(\pi/2-\beta)}+e^{i(-\pi/4+\beta)}}{2} . $$ This complex number should have modulus $1$ and argument $\alpha$. Solving polynomial equation $w\overline{w} = 1$ for $e^{i\beta}$, I get four solutions; $\tan \beta$ is a zero of $7X^4+24X^3-10X^2+8X-1$ the one that matches the picture is such that $\tan \beta \approx 0.14$ and $\beta \approx 8.03^\circ$. Putting this in the expression $w$, we take its argument to get $\alpha$. The result says $\tan \alpha\approx 0.27$.
The angle between the whole thing (which has the big lake) and the middle red part (which has the second lake) is $\gamma := \pi/2-\beta-\alpha$. Using the addition formula for tangents, I get $\tan \gamma$ as a zero of $49X^4-254X^2-47$. The zero that we want is $$ \frac{\sqrt{127+96\sqrt{2}\;}}{7} $$ and $\delta$ is its arctangent.
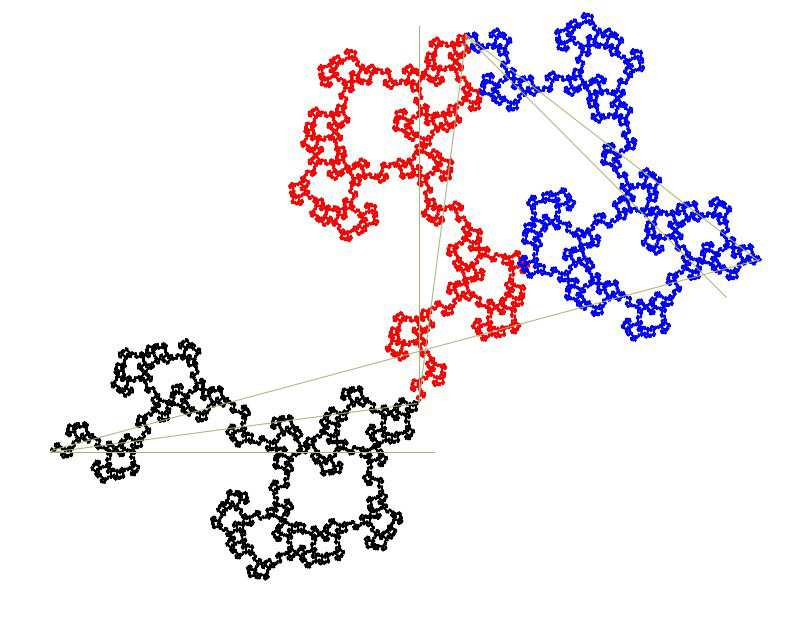
So, to verify the $66$ degree answer: Here is a large picture on the right, then the same picture rotated $66$ degrees and shrunk by fator $1/2$ on the left. Two of the lakes are colored to help comparing them (for size and orientation).