Consider a $\mathbb{Z}^d$ lattice whose edges connect nearest-neighbor sites only, i.e. a $d$-dimensional hypercubic grid. Let $C$ be a closed curve along such edges. In general, for $d>2$ such curves are non-planar. If an elementary square is given area 1, is there any formula that computes the minimal area bounded by a generic lattice curve $C$? For example, the following curve has minimal area of 2:
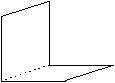
Edit: Precisely, what I would like to know is if it is possible to decide whether a generic curve $C$ bounds an even or odd area. For example, the curve above has area 2, which is even. I suspect that "eveness"parity is a property of the curve and is preserved in non-minimal areas, but I don't know how to prove it.
