First, we note that Stirling's series yields $4^{-k} \binom{2k}{k} = \frac{1}{\sqrt{\pi k}}e^{-1/8k + O(k^{-3})}$.
Let $c = k/n$. Then
- $\log n!$ expands as $n \log n - n + O(\log n)$.
- $\log (2k)!$ expands as $2cn \log 2cn - 2cn + O(\log n)$.
- $\log(n-2k)!$ expands as $n(1-2c)(\log n - \log(1-2c)) - n(1-2c) + O(\log n)$$n(1-2c)(\log n + \log(1-2c)) - n(1-2c) + O(\log n)$.
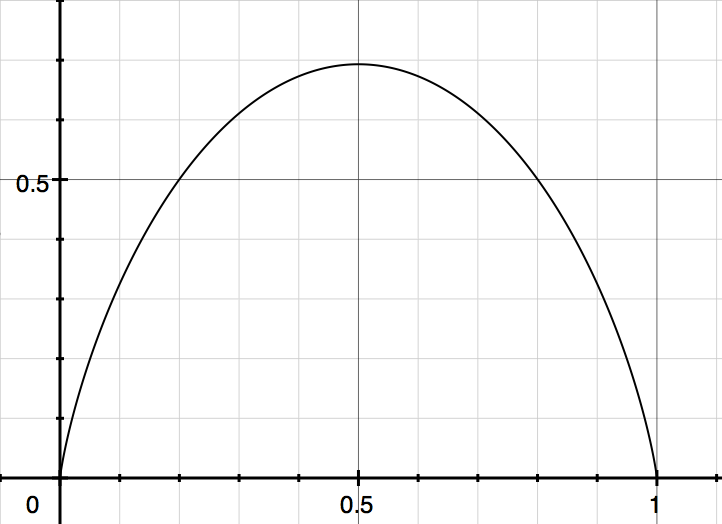
- $\log \binom{n}{2k}$ expands as $-2cn\log 2c + n(1-2c) \log (1-2c) + O(\log n)$$-2cn\log 2c - n(1-2c) \log (1-2c) + O(\log n)$. This is $n$ times the following function of $c$$2c$:

- $\frac{2n \log 2}{\log n} \log\left(\frac{1}{\sqrt{\pi k}}e^{-1/8k + O(k^{-3})}\negthinspace\right)$ expands as $\frac{n \log 2}{\log n}(-\log n -\log c -\log \pi) - O(\frac{1}{c\log n})$
We need to consider the asymptotics of the sum of the 4th and 5th terms. The 5th term is dominated by $-n \log 2$, unless $c\sim 1/n$ (i.e., $k\sim 1$), while the graph suggests that the fourth term is dominated by $n$ times roughly $0.15$. We can make this more precise by taking- a derivative with respectshort calculation shows we don't need to $c$: solving $2c(1-2c)=e^{-2}$ yields $c$worry about this case). From calculus $0.0806891^+$, with optimal(or brief examination of Pascal's triangle) the fourth term achieves its maximum value aboutof $0.14676^+$$n \log 2$ at $c=1/4$. Each term in the The sum is then has logarithm at most aboutdominated by the term $-0.5463^+ n$ for nontrivial$\frac{n \log 2}{\log n}(-\log c -\log \pi)$. Since $k$$\pi c = \pi/4 < 1$, andwe have $0$ for$(-\log c -\log \pi)>0$, so the sum $k\sim 0$$\frac{n \log 2}{\log n}(-\log c -\log \pi) + O(\log n)$ increases without bound. Thus
In conclusion, the sum is eventually bounded above by $1+\frac{n}{2} e^{-0.54 n}$ which converges todiverges, because the summand for $1$$k = \lfloor \frac{n}{4} \rfloor$ increases without bound as $n \to +\infty$$n$ increases.

